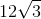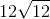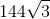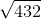Division of square roots is easy, since you can combine the roots and treat it like any other fraction. Thus, you can convert our fraction as follows:

\(\displaystyle \small \frac{\sqrt{1155}}{\sqrt{560}} = \sqrt{\frac{1155}{560}}\)

Next, you begin to reduce the fraction:

\(\displaystyle \small \small \sqrt{\frac{1155}{560}} = \sqrt{\frac{3*5*7*11}{2*2*2*2*5*7}}\)

This reduces to:

\(\displaystyle \small \sqrt{\frac{3*11}{2*2*2*2}}\)

Now, break this apart again into:

\(\displaystyle \small \frac{\sqrt{33}}{\sqrt{16}}\), which is \(\displaystyle \small \frac{\sqrt{33}}{4}\)

√75 can be broken down to √25 * √3. Which simplifies to 5√3.

Rewrite what is under the radical in terms of perfect squares:

\(\displaystyle x^{2}=x\cdot x\)

\(\displaystyle x^{4}=x^{2}\cdot x^{2}\)

\(\displaystyle x^{6}=x^{3}\cdot x^{3}\)

Therefore, \(\displaystyle \sqrt{a^{3}b^{4}c^{5}}= \sqrt{a^{2}a^{1}b^{4}c^{4}c^{1}}=ab^{2}c^{2}\sqrt{ac}\).

We know that 25 is a factor of 50. The square root of 25 is 5. That leaves \(\displaystyle \sqrt{2}\) which can not be simplified further.

Multiply by the conjugate and the use the formula for the difference of two squares:

\(\displaystyle \frac{x + \sqrt{3}}{3x + \sqrt{2}}\)

\(\displaystyle =\) \(\displaystyle \frac{x + \sqrt{3}}{3x + \sqrt{2}}\cdot \frac{3x - \sqrt{2}}{3x - \sqrt{2}}\)

\(\displaystyle =\) \(\displaystyle \frac{3x^{2} -x \sqrt{2} + 3x\sqrt{3} - \sqrt{6}}{(3x)^{2} - (\sqrt{2})^{2}}\) 

\(\displaystyle =\) \(\displaystyle \frac{3x^{2} -x \sqrt{2} + 3x\sqrt{3} - \sqrt{6}}{9x^{2} - 2}\)

First find all of the prime factors of \(\displaystyle 468\)

\(\displaystyle 468=6\ast78=6\ast6\ast13=2\ast3\ast2\ast3\ast13\)

So \(\displaystyle \sqrt{468}=\sqrt{2\ast2\ast3\ast3\ast13}=2\ast3\sqrt{13}=6\sqrt{13}\)

\(\displaystyle \sqrt{432}\)

1. We know that \(\displaystyle 432=(144)(3)\), which we can separate under the square root:

\(\displaystyle \sqrt{144\cdot 3}\)

2. 144 can be taken out since it is a perfect square: \(\displaystyle 12\cdot12=144\). This leaves us with:

\(\displaystyle 12\sqrt{3}\)

This cannot be simplified any further.

When simplifying square roots, begin by prime factoring the number in question. For \(\displaystyle \small 420\), this is:

\(\displaystyle \small 420 = 2*2*3*5*7\)

Now, for each pair of numbers, you can remove that number from the square root. Thus, you can say:

\(\displaystyle \small \small \sqrt{420}=2\sqrt{105}\)

Another way to think of this is to rewrite \(\displaystyle \small \small \sqrt{420}\) as \(\displaystyle \small \sqrt{4}*\sqrt{105}\). This can be simplified in the same manner.

When simplifying square roots, begin by prime factoring the number in question. This is a bit harder for \(\displaystyle \small 700700\). Start by dividing out \(\displaystyle \small 100\):

\(\displaystyle \small 700700 = 7007 * 100\)

Now, \(\displaystyle \small 7007\) is divisible by \(\displaystyle 7\), so:

\(\displaystyle \small \small 700700 =1001 * 7 * 2*2*5*5\)

\(\displaystyle \small 1001\) is a little bit harder, but it is also divisible by \(\displaystyle \small 7\), so:

\(\displaystyle \small 700700 =143*7 * 7 * 2*2*5*5\)

With some careful testing, you will see that \(\displaystyle \small 143 = 11 * 13\)

Thus, we can say:

\(\displaystyle \small \small 700700 = 2*2*5*5*7 * 7*11*13\)

Now, for each pair of numbers, you can remove that number from the square root. Thus, you can say:

\(\displaystyle \small \small \sqrt{700700} = 2*5*7*\sqrt{143}=70\sqrt{143}\)

Another way to think of this is to rewrite \(\displaystyle \small \small \small \sqrt{700700}\) as \(\displaystyle \small \sqrt{4} * \sqrt{25} * \sqrt{49} * \sqrt{143}\). This can be simplified in the same manner.

To simplify a square root, you have to factor the number and look for pairs. Whenever there is a pair of factors (for example two twos), you pull one to the outside.

Thus when you factor 96 you get
\(\displaystyle \\ \sqrt{96}=\sqrt{2\cdot 2\cdot 2\cdot2\cdot2\cdot 3}\\ \\=2\cdot 2\sqrt{2\cdot 3}\\ \\=4\sqrt{6}\)