ACT Math : How to find an angle in a hexagon
Study concepts, example questions & explanations for ACT Math
All ACT Math Resources
Example Questions
Example Question #1 : How To Find An Angle In A Hexagon
The sum of all the angles inside of a regular hexagon is 
In a regular hexagon, all of the sides are the same length, and all of the angles are equivalent. The problem tells us that all of the angles inside the hexagon sum to 
Example Question #2 : How To Find An Angle In A Hexagon
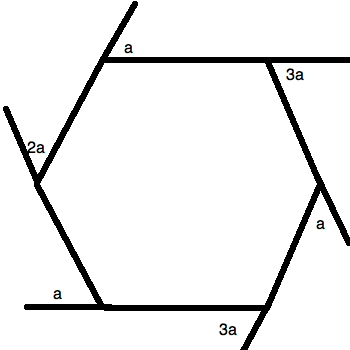
All of the angles marked are exterior angles.
What is the value of 
There are two key things for a question like this. The first is to know that a polygon has a total degree measure of:
Therefore, a hexagon like this one has:
Next, you should remember that all of the exterior angles listed are supplementary to their correlative interior angles. This lets you draw the following figure:
Now, you just have to manage your algebra well. You must sum up all of the interior angles and set them equal to 
Simplify and solve for 
This is 
Example Question #1 : How To Find An Angle In A Hexagon
The figure above is a hexagon. All of the angles listed (except the interior one) are exterior angles to the hexagon's interior angles.
What is the value of 
There are two key things for a question like this. The first is to know that a polygon has a total degree measure of:
Therefore, a hexagon like this one has:
Next, you should remember that all of the exterior angles listed are supplementary to their correlative interior angles. This lets you draw the following figure:
Now, you just have to manage your algebra well. You must sum up all of the interior angles and set them equal to 
Solve for 
Certified Tutor
All ACT Math Resources
