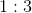The net force on the electron is the sum of the forces between the electron and each of the protons:

$\displaystyle F_{net}=F_1+F_2$

These forces are given by Coulomb's law:

$\displaystyle F=k\frac{q_1q_2}{r^2}$

Using the numbers given, we get:

$\displaystyle F_1=(8.99\cdot10^9)\frac{(+1.60 \cdot 10^{-19})(-1.60 \cdot 10^{-19})}{(30 \cdot 10^-6)^2} = 2.56 \cdot 10^{-19} N$

$\displaystyle F_2=(8.99\cdot10^9)\frac{(+1.60 \cdot 10^{-19})(-1.60 \cdot 10^{-19})}{(10 \cdot 10^-6)^2} = 2.30 \cdot 10^{-18} N$

Because opposite charges attract, $\displaystyle F_1$ points left (the negative direction) and $\displaystyle F_2$ points right (the positive direction).

Therefore, the net force is

$\displaystyle F_{net} = -2.56 \cdot 10^{-19} N + 2.30 \cdot 10^{-18} N = 2.04 \cdot 10^{-18}N$

Because this value is positive, the direction is rightward.

This question is very simple if you realize that the force experienced by both charges is equal.

The definition of the two electrostatic forces are given by Coulomb's law:

$\displaystyle F=-k\frac{q_1q_2}{r^2}$

In this question, we can rewrite this equation in terms of our given system.

$\displaystyle F_a = -k\frac{q_a q_b}{r^2}=-k\frac{(0.003C)(0.006C)}{(1.5m)^2}=-k(8*10^{-6}\frac{C^2}{m^2})$

$\displaystyle F_b = -k\frac{q_b q_a}{r^2}=-k\frac{(0.006C)(0.003C)}{(1.5m)^2}=-k(8*10^{-6}\frac{C^2}{m^2})$

It doesn’t matter if the charges of the two particles are different; both particles experience the same force because the charges of both particles are accounted for in the electrostatic force equation (Coulomb's law). This conclusion can also be made by considering Newton's third law: the force of the first particle on the second will be equal and opposite the force of the second particle on the first.

Since the forces are equal, their ratio will be $\displaystyle \small 1:1$.

Two principal realizations help with solving this problem, both derived from Gauss’ law for electricity:

1) The excess charge on an ideal conducting sphere is uniformly distributed over its surface

2) A uniform shell of charge acts, in terms of electric force, as if all the charge were contained in a point charge at the sphere’s center

With these realizations, an application of Coulomb’s law answers the question. If $\displaystyle q_{2}$ is the point charge outside the sphere, then the force $\displaystyle F_{q2}$ on $\displaystyle q_{2}$ is:

$\displaystyle F_{q2} = \frac{kq_{sphere}q_{2}}{r^{2}}$

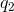
In this equation, $\displaystyle k$ is Coulomb’s constant, _{$\displaystyle q_{sphere}$}is the excess charge on the spherical conductor, and $\displaystyle r$ is total distance in meters of $\displaystyle q_{2}$ from the center of the conducting sphere.

$\displaystyle r=0.20m+0.30m=0.50m$

Using the given values in this equation, we can calculate the generated force:

$\displaystyle F_{q2}=\frac{(8.99*10^9\frac{m^2N}{C^2})(10*10^{-6}C)(5*10^{-6}C)}{(0.5m)^2}$

$\displaystyle F_{q2}=\frac{0.4495m^2N}{0.25m^2}=1.798N$

Coulomb's law gives the relationship between the force of an electric field and the distance between two charges:

$\displaystyle F_e=k\frac{q_1q_2}{r^2}$

The strength of the force will be inversely proportional to the square of the distance between the charges.

When the distance between the charges is doubled, the total force will be divided by four (quartered).

$\displaystyle F_e=k\frac{q_1q_2}{(2r)^2}=k\frac{q_1q_2}{4r^2}=\frac{1}{4}(k\frac{q_1q_2}{r^2})$

Use Coulomb's Law

$\displaystyle F=\frac{kq_1q_2}{r^2}$

Plug in known values and solve.

$\displaystyle \frac{(9\cdot10^{9})(10\cdot10^{-6})(-20\cdot10^{-6})}{0.5^{2}}$

$\displaystyle F=-7.2N$

A negative value for electric force indicates an attractive force. This makes sense since our two charges have opposite signs. Since we're asked for magnitude, all answer choices are positive.

Use Coulomb's law.

$\displaystyle F=\frac{kq_1q_2}{r^2}$

Plug in known values and solve.

$\displaystyle F=\frac{(9\cdot10^{9})(-12\cdot10^{-6})(-13\cdot10^{-6})}{(0.14^{2})}$

$\displaystyle F=71.63N$

Note that this force is positive, which means it's repulsive.

Use Coulomb's law.

$\displaystyle F=\frac{kq_1q_2}{r^2}$

Plug in known values and solve.

$\displaystyle F=\frac{(9\cdot10^{9})(15\cdot10^{-6})(20\cdot10^{-6})}{(10^{2})}$

$\displaystyle F=0.027N$

Note that this force is positive, which means that it's repulsive.

Use Coulomb's law.

$\displaystyle F=\frac{kq_1q_2}{r^2}$

Plug in known values and solve.

$\displaystyle F=\frac{(9\cdot10^{9})(15\cdot10^{-6})(15\cdot10^{-6})}{(0.15^{2})}$

$\displaystyle F=90N$

Note that the force between two charges of the same sign (both positive or both negative) is positive. This indicates the force is repulsive, which makes sense since both charges are positive.

The force of attraction/repulsion between two point charges is given by Coulomb's Law:

$\displaystyle F=k\frac{|q_1||q_2|}{r^2}$

If the charges are of like sign, then there well be a repulsive force between the two. Alternatively, if the net force is positive, it is repulsive; if it is negative, it is attractive.

Therefore, the force of repulsion between the two charges is:

$\displaystyle F=8.9876\cdot10^9\frac{Nm^2}{C^2}\cdot\frac{60\mu C(150\mu C)}{(3m)^2}$

$\displaystyle F=8.9876\cdot10^9\frac{Nm^2}{C^2}\cdot\frac{60\cdot10^{-6} C(150\cdot10^{-6} C)}{(3m)^2}$

$\displaystyle F=8.9876\cdot10^9\cdot\frac{Nm^2}{C^2}\cdot\frac{9000\cdot10^{-12} C^2)}{9m^2}$

$\displaystyle F=8.9876N$

The force of attraction/repulsion between two point charges is given by Coulomb's Law:

$\displaystyle F=k\frac{|q_1||q_2|}{r^2}$

If the charges are of like sign, then there well be a repulsive force between the two.

Work is given as the dot product of force and distance. However, in this case, force is also dependent on distance.

The amount of work required to move a charge an incremental distance, $\displaystyle dr$, is given as:

$\displaystyle dW=-k\frac{q_1q_2}{r^2}dr$

The negative sign in this case is to account for repulsion.

The total work to change distances between charges can then be found by taking the integral with respect to distance:

$\displaystyle W=\int_{d_1}^{d^2} -k\frac{q_1q_2}{r^2}dr$

Since $\displaystyle k,q1,q2$ are constants, they can be factored out of the integral:

$\displaystyle W=-kq_1q_2\int_{d_1}^{d_2} \frac{1}{r^2}dr$

$\displaystyle W=kq_1q_2\frac{1}{r}|_{d_1}^{d_2}$

$\displaystyle W=(8.9876\cdot10^9\frac{Nm^2}{C^2})(60\cdot10^{-6}C)(150\cdot10^{-6}C) \left(\frac{1}{2m}-\frac{1}{3m}\right)$

$\displaystyle W=13.48J$