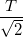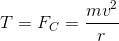To find the equation for velocity, we take the first derivative of the position function. Don't forget the chain rule for the inside of the cosine!

\(\displaystyle v=\frac{d(x)}{dt}\)

\(\displaystyle v=(-2.00\pi \frac{m}{s})sin(\pi t+\frac{\pi }{2})\)

\(\displaystyle v=(-2.00\pi \frac{m}{s})sin(\pi (2.00s))+\frac{\pi }{2})\)

\(\displaystyle v=2\pi \frac{m}{s}\)

To solve this problem, we can use the following equation:

\(\displaystyle \small T=2\pi\sqrt{\frac{m}{k}}\)

Here, \(\displaystyle T\) is the period of an oscillation, \(\displaystyle m\) is the mass of the oscillating thing in kg, and \(\displaystyle k\) is the spring constant in \(\displaystyle \frac{kg}{s^2}\).

Rearranging the equation to solve for the spring constant, \(\displaystyle k\), we get:

\(\displaystyle k\cdot =\frac{m}{(\frac{T}{2\pi})^2}\)

Plugging in the two known values, we get:

\(\displaystyle k\cdot =\frac{0.300kg}{(\frac{2.27s}{2\pi})^2}\)

Solving for \(\displaystyle k\), we get \(\displaystyle 0.575\frac{kg}{s^2}\).

Assuming this is a frictionless table, we don't have to take the work done by friction into account.

This is a conservation of energy problem. In this problem we have to realize that the potential energy of the spring at \(\displaystyle 7\:cm\) is equal to the kinetic energy of the spring at \(\displaystyle 3\:cm\)+ the potential energy of the spring at \(\displaystyle 3\:cm\).

This can be shown in the following equation:

\(\displaystyle \frac{1}{2}kx_7^2 = \frac{1}{2}mv^2 + \frac{1}{2}kx_3^2\)

If we solve for \(\displaystyle v\), we get the following equation:

\(\displaystyle v = \sqrt{2\cdot\frac{\frac{1}{2}kx_7^2-\frac{1}{2}kx_3^2}{m}}\)

Remember to convert the distances given in centimeters to meters.

If we plug in all the variables, we get \(\displaystyle v = 1.1\:m/s\)

The correct answer is \(\displaystyle 0^\circ\). Since the pendulum is at the bottom of its motion at this point, it has the lowest amount of energy given to gravitational potential and thus, the highest kinetic energy. 

Use the equation for the period of a simple pendulum:

\(\displaystyle T=2\pi\sqrt\frac{L}{g}\) 

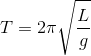
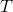
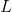
Here, \(\displaystyle T\) is the period in seconds, \(\displaystyle L\) is the length of the pendulum in meters, and \(\displaystyle g\) is the acceleration due to gravity in \(\displaystyle \frac{m}{s^2}\).

We can plug in the given quantities for length and acceleration to solve for the period.

\(\displaystyle T=2\pi\sqrt\frac{1}{9.8}\approx2s\)

Note that the mass of the rope as negligible. We also need not incorporate the extraneous information regarding the weight of the steel ball attached to the pendulum. This does not influence the period of this simple pendulum.

Because the springs are effectively in a parallel arrangement already, moving one does not change the effective spring constant, and therefore does not affect the period.

This is a centripetal force problem. In this case the tension on the rope is the centripetal force that keeps the ball moving on a circle.

\(\displaystyle T=F_C=\frac{mv^2}{r}\)

If we want for the rope not to break, then the tension should never exceed \(\displaystyle T_{max}\).

\(\displaystyle T_{max}\geq\frac{mv^2}{r}\)

Now we just solve for velocity:

\(\displaystyle v\leq\sqrt{\frac{rT_{max}}{m}}}\)

The correct answer is moment of inertia. For linear equations, mass is what resists force and causes lower linear accelerations. Similarly, in rotational equations, moment of inertia resists torque and causes lower angular accelerations. 

Just as force causes linear acceleration, torque causes angular acceleration. This can be seen most in the linear-rotational comparison of Newton's second law:

\(\displaystyle F=ma\)

\(\displaystyle \tau=I\alpha\)

Linear (tangential) velocity, \(\displaystyle v_{T}\) is given by the following equation: 

\(\displaystyle v_{T}=\omega r\)

Here, \(\displaystyle \omega\) is the angular velocity in radians per second and \(\displaystyle r\) is the radius in meters.

\(\displaystyle r=1m\)

Solve.

\(\displaystyle \omega = 30\pi \frac{rad}{s}\)

 \(\displaystyle \omega = 30\pi\frac{m}{s}\)