We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 1 : Functions

Study concepts, example questions & explanations for Calculus 1

Create An Account

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2807 : Calculus

A toy car is thrown straight upward into the air. The equation of the position of the object is:

$y(t) = t^{2} - 5t$

What is the instantaneous velocity of the toy car at t = 2 seconds?

Possible Answers:

$4\tfrac{m}{s}$

$6 \tfrac{m}{s}$

none of the answers

$1\tfrac{m}{s}$

$2 \tfrac{m}{s}$

Correct answer:

none of the answers

Explanation:

To find the velocity of the toy car, we take the derivative of the position equation. The velocity equation is

v(t) = 2t - 5

Then we insert t = 2 seconds to find the instantaneous velocity. A negative instantaneous velocity mean that the toy is slowing down.

Report an Error

Example Question #52 : How To Find The Meaning Of Functions

Find dv/dt if:

$v=\sin(2x);x=2t^2-5t+9$

Possible Answers:

$(4t-5)\cos(4t^2+5t-9)$

$(4t+5)\sin(2t^2-5t+9)$

$(4t^2-10t+18)\cos(2t)$

$(8t+10)\cos(4t^2-10t-18)$

$(8t-10)\cos(4t^2-10t+18)$

Correct answer:

$(8t-10)\cos(4t^2-10t+18)$

Explanation:

Solving for dv/dt, requires use of the chain rule:

$\frac{dv}{dt}=\frac{dv}{dx}\frac{dx}{dt}=\frac{d}{dx}[\sin(2x)]\frac{d}{dt}[2t^2-5t+9]$

$\frac{dv}{dt}=(2\cos(2x))(4t-5)=(4t-5)(2\cos(2(2t^2-5t+9)))$

$\frac{dv}{dt}=(8t-10)\cos(4t^2-10t+18)$

This is one of the answer choices.

Report an Error

Example Question #51 : How To Find The Meaning Of Functions

Find

$\lim_{x\rightarrow 1} \frac{6x^2-7x+1}{x^3-1}$ .

Possible Answers:

$-\infty$

$+\infty$

$\frac{5}{3}$

Undefined

Correct answer:

$\frac{5}{3}$

Explanation:

If you plug into the equation, you get $\frac{0}{0}$ , meaning you should use L'Hopital's Rule to find the limit.

L'Hopital's Rule states that if

$\lim_{x \to c}f(x)=\lim_{x \to c}g(x)=0 \text{ or } \pm\infty$ and that if

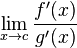$\lim_{x\to c}\frac{f'(x)}{g'(x)}$ exists,

then

$\lim_{x\to c}\frac{f(x)}{g(x)} = \lim_{x\to c}\frac{f'(x)}{g'(x)}$

In this case:

f(x) = 6x^2 -7x+1

g(x) = x^3-1

Start by finding the derivative of the numerator and evaluating it at :

$\frac{\mathrm{d} }{\mathrm{d} x}6x^2-7x + 1=12x-7=5$

Do the same for the denominator:

$\frac{\mathrm{d} }{\mathrm{d} x}x^3-1 = 3x^2 = 3$

The limit is then equal to the derivative of the numerator evaluated at divided by the derivative of the denominator evaluated at , or $\frac{5}{3}$ .

Report an Error

Example Question #61 : How To Find The Meaning Of Functions

Find the slope of the tangent line equation for $y = \sin (2x) \cdot x^2$ when $x = \frac{\pi}{2}$ .

Possible Answers:

$-2 \pi$

$- \frac{\pi^2}{4}$

$- \frac{\pi^2}{2}$

$\pi$

Correct answer:

$- \frac{\pi^2}{2}$

Explanation:

To find the slope, we need to differentiate the given function. By product rule and chain rule, we have $y' = 2 \cos (2x) \cdot x^2 + \sin (2x) \cdot (2x).$ $\Rightarrow y'(\frac{\pi}{2}) = 2 \cos {\pi} \cdot \frac{\pi^2}{4} + \sin \pi \cdot \pi \\ = 2 \cdot (-1) \cdot \frac{\pi^2}{4} + 0 \cdot \pi = - \frac{\pi^2}{2} .$

Report an Error

Example Question #1781 : Functions

Function

What is a function?

Possible Answers:

None of the above

A function is a matematical equation with one or, more variables

A function is an equation with at least two unknowns such as x and y

A function is a relationship that assigns to each input value a single output value

A function is a relationship that can produce multiple output values for each single input value

Correct answer:

A function is a relationship that assigns to each input value a single output value

Explanation:

You could think of a function as a machine that takes in some number, performs an operation on it, and then spits out another number

Report an Error

Example Question #2811 : Calculus

Identify Function

Which one of the following is not a function?

Possible Answers:

$f(x)=\sqrt{x}(\sqrt{x}-x\sqrt{x})$

$f(x)=5x^{5}+2$

$ax^{2}+bx+c=y$

$y^{2}=x+1$

$19x^{2}+157x-\frac{5}{2}=y$

Correct answer:

$y^{2}=x+1$

Explanation:

For any value of in $y^{2}=x+1$ , there will be two values of . So it is not a function.

Report an Error

Example Question #1783 : Functions

Type of Functions

What type of function is this:

$f(x)=\begin{Bmatrix} x^2+1 & if \, \, x\leqslant 3\\ 12-x& if \, \, x>3\end{Bmatrix}$

Possible Answers:

Quadratic

Rational

Polynomial

Piecewise

Linear

Correct answer:

Piecewise

Explanation:

Piecewise functions are a special type function in which the formula changes for different values.

Report an Error

Example Question #65 : How To Find The Meaning Of Functions

Find the domain of the function $f(x) = \frac {\ln x}{\sqrt {x^2-1}}.$

Possible Answers:

0 < x < 1

x > 0 or x < -1

x>1

-1 < x <1

x>1 or x < -1

Correct answer:

x>1

Explanation:

$\ln(x)$ is defined when x>0 , and $\sqrt {x^2-1}$ is defined when $x^2-1 \geq 0$ . Since the radical part is in the denominator, it cannot be . Therefore, we need $x^2 -1 >0 \Rightarrow x>1 \or \ x<-1.$ Combining this domain with x>0 , we get x>1 .

Report an Error

Example Question #66 : How To Find The Meaning Of Functions

$f(x)= 3x^{3}+7x-2$

What is the slope of this curve at x=4 ?

Possible Answers:

152

150

151

220

218

150

152

151

218

220

Correct answer:

151

Explanation:

To find the slope of a curve at a certain point, you must first find the derivative of that curve.

The derivative of this curve is $f'(x)=9x^{2}-7$ .

Then, plug in for into the derivative and you will get 151 as the slope of f(x) at x=2 .

Report an Error

Example Question #66 : How To Find The Meaning Of Functions

What are the critical points of f(x)=x^3-2x^2+x ?

Possible Answers:

x= 1

$x=\frac{1}{3}, -1$

$x=\frac{1}{3}$

$x=\frac{1}{3}, 1$

Correct answer:

$x=\frac{1}{3}, 1$

Explanation:

To find the critical points of a function, you need to first find the derivative and then set that equal to 0. To find the derivative, multiply the exponent by the leading coefficient and then subtract 1 from the exponent. Therefore, the derivative is $f{}'(x)=3x^2-4x+1$ . Set that equal to 0 and then factor so that you get: (3x-1)(x-1)=0 . Solve for x in both expressions so that your answer is: $x=\frac{1}{3}, 1$ .

Report an Error

View Calculus Tutors

Divyansh
Certified Tutor

Dalhousie University, Bachelor of Technology, Chemical Engineering.

View Calculus Tutors

Naomi
Certified Tutor

Earlham College, Bachelor in Arts, Mathematics. Saint Josephs University, Master of Science, Education.

View Calculus Tutors

Magdy
Certified Tutor

Cairo University, Egypt, Bachelor of Science, Electrical Engineering. New Mexico State University-Main Campus, Doctor of Phil...

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in New York City, Physics Tutors in Boston, Statistics Tutors in New York City, GMAT Tutors in Atlanta, Math Tutors in Atlanta, English Tutors in Houston, Biology Tutors in Philadelphia, Statistics Tutors in Boston, MCAT Tutors in Houston, LSAT Tutors in Houston

Popular Courses & Classes

ISEE Courses & Classes in Boston, MCAT Courses & Classes in Phoenix, GRE Courses & Classes in Dallas Fort Worth, GRE Courses & Classes in Phoenix, MCAT Courses & Classes in Atlanta, LSAT Courses & Classes in Atlanta, ACT Courses & Classes in Chicago, ISEE Courses & Classes in Seattle, GRE Courses & Classes in New York City, GMAT Courses & Classes in Denver

Popular Test Prep

SAT Test Prep in Denver, SAT Test Prep in San Francisco-Bay Area, SAT Test Prep in Dallas Fort Worth, LSAT Test Prep in Los Angeles, MCAT Test Prep in Atlanta, SAT Test Prep in Seattle, SAT Test Prep in Houston, ACT Test Prep in San Francisco-Bay Area, MCAT Test Prep in Chicago, MCAT Test Prep in Philadelphia

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 1 : Functions

Study concepts, example questions & explanations for Calculus 1

All Calculus 1 Resources

Example Questions

Example Question #2807 : Calculus

Example Question #52 : How To Find The Meaning Of Functions

Find dv/dt if:

Example Question #51 : How To Find The Meaning Of Functions

Example Question #61 : How To Find The Meaning Of Functions

Example Question #1781 : Functions

Example Question #2811 : Calculus

Example Question #1783 : Functions

Example Question #65 : How To Find The Meaning Of Functions

Example Question #66 : How To Find The Meaning Of Functions

Example Question #66 : How To Find The Meaning Of Functions

All Calculus 1 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: