is a degree 3 polynomial, so we don't have any easy formulas for calculating possible roots--we just have to check individual values to see if they work. We can use the rational root test to narrow the options down. Remember, if we have a polynomial of the form  then any rational root will be of the form p/q where p is a factor of  and q is a factor of . Fortunately in this case,  so we only need to check the factors of , which is -15. Let's start with the easiest one: 1.

 It doesn't work.

If we try the next number up, 3, we get this:

 It worked! So we know that a factor of our polynomial is . We can divide this factor out:

and now we need to see if  has any roots. We can actually solve quadratics so this is easier.

There aren't any real numbers that square to get -5 so this has no roots. Thus,  only has one root.

As a polynomial function, the graph of  is continuous. By the Intermediate Value Theorem, if  or , then there must exist a value  such that . 

Set  and . It is not true that , so the Intermediate Value Theorem does not prove that there exists  such that . However, it does not disprove that such a value exists either. For example, observe the graphs below:

Both are polynomial graphs fitting the given conditions, but the only the equation graphed at right has a zero on .

One way to answer this question is as follows:

Let . By a corollary of the Factor Theorem,  is divisible by  if and only if the sum of its coefficients (accounting for minus symbols) is 0.  has 

as its coefficient sum, so  is indeed divisible by .

Let . By a corollary of the Factor Theorem,  is divisible by  if and only if the alternating sum of its coefficients (accounting for minus symbols) is 0.

To find this alternating sum, it is necessary to reverse the symbol before all terms of odd degree. In , there is one such terms, the  term, so the alternating coefficient sum is  

,

so  is not divisible by .

As a polynomial function, the graph of  is continuous. By the Intermediate Value Theorem, if  or , then there must exist a value  such that . 

Setting , and looking at the second condition alone, this becomes: If , then there must exist a value  such that  - that is,  must have a zero on . The conditions of this statement are met , since   - and  - so  does have a zero on this interval.

By the Factor Theorem,  is a factor of a polynomial  if and only if . It is given that  is a factor of , so it follows that .

 is an even function, so, by definition, for all  in its domain, . Setting , ; by substitution, . It follows that  is also a factor of , making the statement true. 

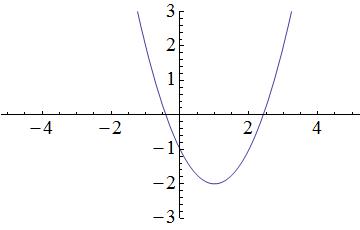
Start by visualizing the graph associated with the function :

Terms within the parentheses associated with the squared x-variable will shift the parabola horizontally, while terms outside of the parentheses will shift the parabola vertically. In the provided equation, 2 is located outside of the parentheses and is subtracted from the terms located within the parentheses; therefore, the parabola in the graph will shift down by 2 units. A simplified graph of  looks like this:

Remember that there is also a term within the parentheses. Within the parentheses, 1 is subtracted from the x-variable; thus, the parabola in the graph will shift to the right by 1 unit. As a result, the following graph matches the given function  :

Factor:

Double check by factoring:

Add together:

Therefore:

1) Split up the middle term so that factoring by grouping is possible.

Factors of 10 include:

1 * 10= 10    1 + 10 = 11

2 * 5 =10      2 + 5 = 7

–2 * –5 = 10    –2 + –5 = –7 Good!

2) Now factor by grouping, pulling "x" out of the first pair and "-5" out of the second.

3) Now pull out the common factor, the "(x-2)," from both terms.

4) Set both terms equal to zero to find the possible roots and solve using inverse operations.

x – 5 = 0,  x = 5

x – 2 = 0, x = 2

To solve for , you need to isolate it to one side of the equation. You can subtract the  from the right to the left. Then you can add the 6 from the right to the left:

Next, you can factor out this quadratic equation to solve for . You need to determine which factors of 8 add up to negative 6:

Finally, you set each binomial equal to 0 and solve for :