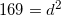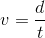GED Science : Motion and Mechanics
Study concepts, example questions & explanations for GED Science
All GED Science Resources
Example Questions
Example Question #1 : Newton's Laws
Which of the following best illustrates Newton's Second Law?
If an object is not accelerating, then the net force on it is zero
A ball is rolled across a table and does not stop until it hits an area with friction
When two cymbals collide, some kinetic energy is converted into sound
As a hammer hits a nail, the nail also exerts a force on the hammer
If an object is not accelerating, then the net force on it is zero
Newton's Second Law is best represented as an equation, in which the product of mass and acceleration is equal to net force:
By this principle, if there is zero acceleration, then the force must also be zero.
A non-zero acceleration must be present if there is a non-zero net force.
Example Question #2 : Newton's Laws
Which of the following is the best example of Newton's Third Law?
A spinning top will not fall unless there is friction with the surface on which it spins
The force of being hit by an object is determined by its mass and acceleration
When two objects collide, the total initial and total final momentum will be equal
If an object's weight rests on the floor, then the floor will exert a normal force on the object
If an object's weight rests on the floor, then the floor will exert a normal force on the object
Newton's Third Law states that for every action there is an equal and opposite reaction. In other words, a force will act in equal and opposite directions on the two objects involved.
Weight and normal force are a specific example of this principle. Acceleration due to gravity creates a downward force, known as weight. This force, however, results in zero downward acceleration when the object is resting on a surface (a book does not fall through a table, for example). For the forces to be in equilibrium, there must be a counteracting upward force. This is known as the normal force, which is equal and opposite to the weight of the object. While weight pulls the object down, normal force pushes it up to create equilibrium.
Example Question #1 : Physics
A car drives miles east and miles north. What is the total displacement of the car?
The car has covered a total distance of miles, however, displacement and distance are not the same thing. Distance is simply the measure of how far the car have moved, regardless of direction. Displacement is a vector quantity, and takes direction into account. Displacement measures the total distance only between the car's starting point and ending point.
To solve this question, it is best to draw a sketch. The resulting sketch will be a right triangle with the hypotenuse equal to the displacement. The Pythagorean Theorem can be used to solve the problem.
Example Question #1 : Motion And Mechanics
A car travels due east for two hours and covers 144km. What is the car's average velocity in meters per second?
This question requires us to convert between units, then solve for the velocity.
First, convert kilometers to meters. There are 1000m in 1km.
Then, convert hours to seconds. There are 60min in 1hr, and 60sec in 1min. In other words, there are 3600s in 1hr.
Now that you have both the distance in meters and the time in seconds, you can find the velocity. Velocity is equal to the distance divided by the time.
Certified Tutor
All GED Science Resources
