All GRE Math Resources
Example Questions
Example Question #3 : Lowest Common Denominator
In simplest form, 
First, find the smallest number that both 


This means that the fist fraction should be multiplied by 

Therefore,

Then, add the numerators while the denomenators stay the same:

Then, reduce the fraction to its lowest terms:

Example Question #61 : Fractions
For how many integers, 


If 





Example Question #1061 : Gre Quantitative Reasoning
Mrs. Lawrence's class has 

In order to find out the probability of choosing a boy, you must first find out how many boys there are. Since there are 


Therefore, the probability of choosing a boy is,

However, this is not one of the answer choices; therefore, you must reduce the fraction.
In order to reduce a fraction, you have to find their GCM, or greatest common multiple. This is the biggest number that will go into both the numerator 



Example Question #3 : Simplifying Fractions
Simplify the fraction:
To simplify the fraction
It may be helpful to write it in terms of scientific notation:
Example Question #1063 : Gre Quantitative Reasoning
Simply the fraction:
To simplify the fraction
Begin by factoring out common terms in the numerator and denominator:
Now notice that the coefficients for the polynomials in the numerator and denominator match the form of Pascal's Triangle:
The fraction can be rewritten as:
And finally reduced to
Example Question #1 : How To Find Out A Mixed Fraction From An Improper Fraction

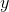


Quantity A:
Quantity B:
Quantity B is greater.
The relationship cannot be determined.
The two quantities are equal.
Quantity A is greater.
The relationship cannot be determined.
Recall that the exponent of the denominator is sutracted from the exponent of the numerator.
Therefore Quantity A is equivalent to x5–y, and because we would then be comparing an arithmetic operation to a geometric operation, it does not matter whether y is a multiple of 5. The two quantities cannot be compared.
Example Question #2 : Mixed / Improper Fractions
Which of the following is the mixed fraction equivalent to 
To begin, notice that using your calculator, you can find:
Now, the closest even multiple of 


This is the same as:


Example Question #12 : Mixed / Improper Fractions
Which of the following is equivalent to 
Although there are many ways to convert improper fractions into mixed fractions, the easiest way is to use your calculator to your advantage. Begin by dividing 










Example Question #1 : How To Find Out A Mixed Fraction From An Improper Fraction
Quantity A:
Quantity B:
Which of the following is true?
Quantity B is larger.
Quantity A is larger.
The two quantities are equal
The relationship of the two quantities cannot be determined based on the information provided.
The two quantities are equal
Though there are several ways you could solve this, let's convert the improper fraction into a mixed one so we can compare them. Start by dividing 


Now, since we know that the two numbers have the same whole-number value, we need to compare their decimal portions. Compare 


Example Question #1525 : Act Math
Write 
To find the improper fraction value, we must effectively add together 71 and 5/7. To do this, we will give 71 a denominator of 7; therefore, we are transforming 71/1 to x/7. The shortest way to do this is to multiply by 7/7 (which really is 1); therefore, 71 = 71 * (7/7) = 497/7.
Now add them: (497 + 5)/7 = 502/7
All GRE Math Resources

































































