We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

GRE Subject Test: Math : Imaginary Numbers & Complex Functions

Study concepts, example questions & explanations for GRE Subject Test: Math

Create An Account

All GRE Subject Test: Math Resources

2 Diagnostic Tests 148 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #292 : Gre Subject Test: Math

Simplify $\frac{i-2}{3-i}$

Possible Answers:

$\frac{2i-5}{5}$

$\frac{10i-2}{7}$

$\frac{i-7}{10}$

$\frac{7-i}{10}$

$\frac{5-2i}{10}$

Correct answer:

$\frac{i-7}{10}$

Explanation:

$\frac{i-2}{3-i}$

In problems like this, you are expected to simplify by removing i from the denominator. To do this, multiply the numerator and denominator by the conjugate of the denominator (switch the sign between the two terms from either a plus to a minus or vice versa) over itself. The conjugate over itself equals 1 and does not change the value of the expression (any number multiplied by 1 is still that number). Multiplying by the conjugate is the only way to eliminate i since there will be no middle term when we foil.

$\frac{i-2}{3-i}\cdot \frac{3+i}{3+i}$

$\frac{(i-2)(3+i)}{(3-i)(3+i)}$

$\frac{3i+i^{2}-6-2i}{9-i^{2}}$

Simplify i squared to be -1 and combine like terms

$\frac{3i+(-1)-6-2i}{9-(-1)}$

$\frac{3i-1-6-2i}{9+1}$

$\frac{i-7}{10}$

Report an Error

Example Question #293 : Gre Subject Test: Math

Simplify $\frac{14i-9}{9+i}$

Possible Answers:

$\frac{54i-18}{25}$

$\frac{23i-11}{65}$

$\frac{-44i+63}{86}$

$\frac{135i-67}{82}$

$\frac{14i+111}{42}$

Correct answer:

$\frac{135i-67}{82}$

Explanation:

$\frac{14i-9}{9+i}$

$\frac{14i-9}{9+i}\cdot \frac{9-i}{9-i}$

$\frac{(14i-9)(9-i)}{(9+i)(9-i)}$

$\frac{126i-81-14i^{2}+9i}{81-i^{2}}$

Simplify i squared to be -1 and combine like terms

$\frac{126i-81-14(-1)+9i}{81-(-1)}$

$\frac{126i-81+14+9i}{81+1}$

$\frac{135i-67}{82}$

Report an Error

Example Question #294 : Gre Subject Test: Math

Simplify $\frac{13i+13}{13i-9}$

Possible Answers:

$\frac{-13+169i}{250}$

$\frac{26-143i}{250}$

$\frac{-13i-169}{-250}$

$\frac{-26+143i}{-125}$

$\frac{26i-143}{125}$

Correct answer:

$\frac{-26+143i}{-125}$

Explanation:

$\frac{13i+13}{13i-9}$

$\frac{13i+13}{13i-9}\cdot \frac{13i+9}{13i+9}$

$\frac{(13i+13)(13i+9)}{(13i-9)(13i+9)}$

$\frac{(169i^{2}+169i+117i+117)}{(169i^{2}-81)}$

Simplify i squared to be -1 and combine like terms

$\frac{(169(-1)+169i+117i+117)}{(169)(-1)-81}$

$\frac{(-169+169i+117i+117)}{(-169-81)}$

$\frac{-52+286i}{-250}$

Each term divides by 2 so make sure to reduce all of the terms

$\frac{-26+143i}{-125}$

Report an Error

Example Question #295 : Gre Subject Test: Math

Simplify $\frac{-12i-17}{6i+11}$

Possible Answers:

$\frac{-72i+13}{99}$

$\frac{24i-15}{81}$

$\frac{-20i-187}{34}$

$\frac{33i-105}{16}$

$\frac{19i+259}{-157}$

Correct answer:

$\frac{19i+259}{-157}$

Explanation:

$\frac{-12i-17}{6i+11}$

$\frac{-12i-17}{6i+11}\cdot \frac{6i-11}{6i-11}$

$\frac{(-12i-17)(6i-11)}{(6i+11)(6i-11)}$

$\frac{-72i^{2}+121i-102i+187}{36i^{2}-121}$

Simplify i squared to be -1 and combine like terms

$\frac{-72(-1)+121i-102i+187}{36(-1)-121}$

$\frac{72+121i-102i+187}{-36-121}$

$\frac{19i+259}{-157}$

Report an Error

Example Question #51 : Imaginary Numbers & Complex Functions

Simplify $\frac{3i+7}{4i-4}$

Possible Answers:

Undefined

$\frac{4i+9}{20}$

$\frac{11i-2}{6}$

$\frac{6i+7}{18}$

$\frac{5i+2}{-4}$

Correct answer:

$\frac{5i+2}{-4}$

Explanation:

$\frac{3i+7}{4i-4}$

$\frac{3i+7}{4i-4}\cdot \frac{4i+4}{4i+4}$

$\frac{(3i+7)(4i+4)}{(4i-4)(4i+4)}$

$\frac{12i^{2}+12i+28i+28}{16i^{2}-16}$

Simplify i squared to be -1 and combine like terms

$\frac{12(-1)+12i+28i+28}{16(-1)-16}$

$\frac{-12+12i+28i+28}{-16-16}$

$\frac{40i+16}{-32}$

Since every term divides by 8 make sure to reduce all the terms by that greatest common factor

$\frac{5i+2}{-4}$

Report an Error

Example Question #52 : Imaginary Numbers & Complex Functions

Simplify

$\frac{5+2i}{-3-2i}$

Possible Answers:

6+i

$\frac{7i+1}{5}$

$\frac{i+2}{10}$

$\frac{-19+4i}{13}$

$\frac{16+4i}{3}$

Correct answer:

$\frac{-19+4i}{13}$

Explanation:

$\frac{5+2i}{-3-2i}$

$\frac{5+2i}{-3-2i}\cdot \frac{-3+2i}{-3+2i}$

$\frac{(5+2i)(-3+2i)}{(-3-2i)(-3+2i)}$

$\frac{-15-6i+10i+4i^{2}}{9-4i^{2}}$

Simplify i squared to be -1 and then combine like terms

$\frac{-15-6i+10i+4(-1)}{9-4(-1)}$

$\frac{-15+4i-4}{9+4}$

$\frac{-19+4i}{13}$

Report an Error

Example Question #111 : Classifying Algebraic Functions

Write the complex number $1-\sqrt{3}i$ in polar form, where polar form expresses the result in terms of a distance from the origin on the complex plane and an angle from the positive -axis, $\theta$ , measured in radians.

Possible Answers:

$\newline r=\sqrt{3} \newline \newline \theta =2 \pi$

$\newline r=\frac{1}{\sqrt{3}} \newline \newline \theta = \frac{3 \pi}{5}$

$\newline r=2 \newline \newline \theta = \frac{5 \pi}{6}$

$\newline r=1 \newline \newline \theta = \frac{\sqrt{3} \pi}{6}$

$\newline r=2 \newline \newline \theta = \sqrt{3} \pi$

Correct answer:

$\newline r=2 \newline \newline \theta = \frac{5 \pi}{6}$

Explanation:

To see what the polar form of the number is, it helps to draw it on a graph, where the horizontal axis is the imaginary part and the vertical axis the real part. This is called the complex plane.

Vecii

To find the angle $\theta$ , we can find its supplementary angle $\Psi$ and subtract it from $\pi$ radians, so $\theta = \pi - \Psi$ .

Using trigonometric ratios, 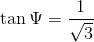$\tan \Psi = \frac{1}{\sqrt{3}}$ and $\arctan({\frac{1}{\sqrt{3}}})= \Psi = \frac{\pi}{6}$ .

Then $\theta = \frac{5\pi}{6}$ .

To find the distance , we need to find the distance from the origin to the point $(-\sqrt{3}, 1)$ . Using the Pythagorean Theorem to find the hypotenuse , $r = \sqrt{ \sqrt{3}^2 + 1^2 }$ or r=2 .

Report an Error

View Tutors

Samuel
Certified Tutor

York University, Bachelor, Physics. York University, Master's/Graduate, Mathematics and Statistics.

View Tutors

Mohammed
Certified Tutor

University of Maryland-College Park, Bachelor of Science, Electrical Engineering. University of Maryland-College Park, Master...

View Tutors

Sumit
Certified Tutor

Calcutta University, Bachelor of Science, Mathematics. Indian Statistical Institute, Master of Science, Mathematics.

All GRE Subject Test: Math Resources

2 Diagnostic Tests 148 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Washington DC, English Tutors in Phoenix, SAT Tutors in Atlanta, Chemistry Tutors in Chicago, Reading Tutors in Denver, Chemistry Tutors in Seattle, Math Tutors in Washington DC, Physics Tutors in San Diego, GRE Tutors in San Francisco-Bay Area, GRE Tutors in Los Angeles

Popular Courses & Classes

GRE Courses & Classes in Phoenix, GMAT Courses & Classes in Houston, ISEE Courses & Classes in Chicago, SSAT Courses & Classes in Denver, LSAT Courses & Classes in Houston, ISEE Courses & Classes in San Francisco-Bay Area, Spanish Courses & Classes in Miami, SAT Courses & Classes in Philadelphia, LSAT Courses & Classes in Denver, ACT Courses & Classes in San Diego

Popular Test Prep

GRE Test Prep in Philadelphia, MCAT Test Prep in Houston, SAT Test Prep in Boston, ISEE Test Prep in New York City, GMAT Test Prep in Houston, MCAT Test Prep in San Diego, ISEE Test Prep in Los Angeles, SSAT Test Prep in Seattle, ACT Test Prep in Los Angeles, SAT Test Prep in San Diego

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

GRE Subject Test: Math : Imaginary Numbers & Complex Functions

Study concepts, example questions & explanations for GRE Subject Test: Math

All GRE Subject Test: Math Resources

Example Questions

Example Question #292 : Gre Subject Test: Math

Example Question #293 : Gre Subject Test: Math

Example Question #294 : Gre Subject Test: Math

Example Question #295 : Gre Subject Test: Math

Example Question #51 : Imaginary Numbers & Complex Functions

Example Question #52 : Imaginary Numbers & Complex Functions

Example Question #111 : Classifying Algebraic Functions

All GRE Subject Test: Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: