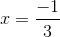Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : Algebra II

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #341 : Algebra Ii

Solve using the quadratic formula:

$\displaystyle 2x^2+2x+3=0$

Possible Answers:

$x= \frac{-1 \pm i\sqrt{5}}{3}$ $\displaystyle x= \frac{-1 \pm i\sqrt{5}}{3}$

$x= \frac{-2 \pm i\sqrt{5}}{3}$ $\displaystyle x= \frac{-2 \pm i\sqrt{5}}{3}$

$x= \frac{-1 \pm i\sqrt{5}}{2}$ $\displaystyle x= \frac{-1 \pm i\sqrt{5}}{2}$

$x= \frac{-3 \pm i\sqrt{5}}{2}$ $\displaystyle x= \frac{-3 \pm i\sqrt{5}}{2}$

$x= \frac{-2 \pm i\sqrt{5}}{2}$ $\displaystyle x= \frac{-2 \pm i\sqrt{5}}{2}$

Correct answer:

$x= \frac{-1 \pm i\sqrt{5}}{2}$ $\displaystyle x= \frac{-1 \pm i\sqrt{5}}{2}$

Explanation:

Use the quadratic formula to solve:

$x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$

a = 2 $\displaystyle a = 2$

b = 2 $\displaystyle b = 2$

c = 3 $\displaystyle c = 3$

$x = \frac{-(2) \pm \sqrt{(2)^2-4(2)(3)}}{2(2)}$ $\displaystyle x = \frac{-(2) \pm \sqrt{(2)^2-4(2)(3)}}{2(2)}$

$x = \frac{-2 \pm \sqrt{-20}}{4}$ $\displaystyle x = \frac{-2 \pm \sqrt{-20}}{4}$

$x = \frac{-2 \pm 2i\sqrt{5}}{4}$ $\displaystyle x = \frac{-2 \pm 2i\sqrt{5}}{4}$

$x = \frac{-1 \pm i\sqrt{5}}{2}$ $\displaystyle x = \frac{-1 \pm i\sqrt{5}}{2}$

Report an Error

Example Question #91 : Intermediate Single Variable Algebra

Solve using the quadratic formula:

$\displaystyle 2a^2-5a+4=0$

Possible Answers:

$a=\frac{4 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{4 \pm i\sqrt{7}}{4}$

$a=\frac{6 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{6 \pm i\sqrt{7}}{4}$

$a=\frac{3 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{3 \pm i\sqrt{7}}{4}$

$a=\frac{7 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{7 \pm i\sqrt{7}}{4}$

$a=\frac{5 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{5 \pm i\sqrt{7}}{4}$

Correct answer:

$a=\frac{5 \pm i\sqrt{7}}{4}$ $\displaystyle a=\frac{5 \pm i\sqrt{7}}{4}$

Explanation:

Use the quadratic formula to solve:

$x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$

a = 2 $\displaystyle a = 2$

b = -5 $\displaystyle b = -5$

c = 4 $\displaystyle c = 4$

$a = \frac{-(-5) \pm \sqrt{(-5)^2-4(2)(4)}}{2(2)}$ $\displaystyle a = \frac{-(-5) \pm \sqrt{(-5)^2-4(2)(4)}}{2(2)}$

$a = \frac{5 \pm \sqrt{-7}}{4}$ $\displaystyle a = \frac{5 \pm \sqrt{-7}}{4}$

$a = \frac{5 \pm i\sqrt{7}}{4}$ $\displaystyle a = \frac{5 \pm i\sqrt{7}}{4}$

Report an Error

Example Question #1 : Using The Quadratic Formula

Solve using the quadratic formula:

$\displaystyle ax^2+bx+3b=0$

Possible Answers:

$x=\frac{-b \pm \sqrt{b^2-12ab}}{2a}$ $\displaystyle x=\frac{-b \pm \sqrt{b^2-12ab}}{2a}$

$x=\frac{-b \pm \sqrt{b^2-8ab}}{2a}$ $\displaystyle x=\frac{-b \pm \sqrt{b^2-8ab}}{2a}$

$x=\frac{b \pm \sqrt{b^2-12ab}}{2a}$ $\displaystyle x=\frac{b \pm \sqrt{b^2-12ab}}{2a}$

$x=\frac{-b \pm \sqrt{b^2-12ab}}{a}$ $\displaystyle x=\frac{-b \pm \sqrt{b^2-12ab}}{a}$

$x=\frac{b \pm \sqrt{b^2-12ab}}{a}$ $\displaystyle x=\frac{b \pm \sqrt{b^2-12ab}}{a}$

Correct answer:

$x=\frac{-b \pm \sqrt{b^2-12ab}}{2a}$ $\displaystyle x=\frac{-b \pm \sqrt{b^2-12ab}}{2a}$

Explanation:

Use the quadratic formula to solve:

$x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$

a = a $\displaystyle a = a$

b = b $\displaystyle b = b$

c = 3b $\displaystyle c = 3b$

$x = \frac{-(b) \pm \sqrt{(b)^2-4(a)(3b)}}{2(a)}$ $\displaystyle x = \frac{-(b) \pm \sqrt{(b)^2-4(a)(3b)}}{2(a)}$

$x = \frac{-b \pm \sqrt{b^2-12ab}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-12ab}}{2a}$

Report an Error

Example Question #1 : Using The Quadratic Formula

Solve using the quadratric formula:

$\displaystyle bx^2+acx+c=0$

Possible Answers:

$x=\frac{-ac\sqrt{a^2c^2-4bc}}{b}$ $\displaystyle x=\frac{-ac\sqrt{a^2c^2-4bc}}{b}$

$x=\frac{-ac\sqrt{a^2c^2-2bc}}{2b}$ $\displaystyle x=\frac{-ac\sqrt{a^2c^2-2bc}}{2b}$

$x=\frac{-ac\sqrt{a^2c^2-4bc}}{2b}$ $\displaystyle x=\frac{-ac\sqrt{a^2c^2-4bc}}{2b}$

$x=\frac{ac\sqrt{a^2c^2-4bc}}{2b}$ $\displaystyle x=\frac{ac\sqrt{a^2c^2-4bc}}{2b}$

$x=\frac{ac\sqrt{a^2c^2-4bc}}{b}$ $\displaystyle x=\frac{ac\sqrt{a^2c^2-4bc}}{b}$

Correct answer:

$x=\frac{-ac\sqrt{a^2c^2-4bc}}{2b}$ $\displaystyle x=\frac{-ac\sqrt{a^2c^2-4bc}}{2b}$

Explanation:

Use the quadratic formula to solve:

$x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$

a = b $\displaystyle a = b$

b = ac $\displaystyle b = ac$

c = c $\displaystyle c = c$

$x = \frac{-(ac) \pm \sqrt{(ac)^2-4(b)(c)}}{2(b)}$ $\displaystyle x = \frac{-(ac) \pm \sqrt{(ac)^2-4(b)(c)}}{2(b)}$

$x = \frac{-ac \pm \sqrt{a^2c^2-4bc}}{2b}$ $\displaystyle x = \frac{-ac \pm \sqrt{a^2c^2-4bc}}{2b}$

Report an Error

Example Question #2 : Quadratic Formula

A baseball that is thrown in the air follows a trajectory of $\displaystyle h(t)= -4t^2 +12t+6$, where h(t) $\displaystyle h(t)$ is the height of the ball in feet and $\displaystyle t$ is the time elapsed in seconds. How long does the ball stay in the air before it hits the ground?

Possible Answers:

Between 3 and 3.5 seconds

Between 3.5 and 4 seconds

Between 2.5 and 3 seconds

Between 2 and 2.5 seconds

Between 4 and 4.5 seconds

Correct answer:

Between 3 and 3.5 seconds

Explanation:

To solve this, we look at the equation h(t)=0 $\displaystyle h(t)=0$.

Setting the equation equal to 0 we get $\displaystyle 0= -4t^2 +12t+6$.

Once in this form, we can use the Quadratic Formula to solve for $\displaystyle t$.

The quadratic formula says that if $\displaystyle 0= -ax^2 +bx+c$, then

$x= \frac{-b\pm\sqrt(b^2-4ac)}{2a}$ $\displaystyle x= \frac{-b\pm\sqrt(b^2-4ac)}{2a}$.

Plugging in our values:

$t= \frac{-12\pm\sqrt{(12^2-4(-4)(6)}}{2(-4)}=\frac{-12\pm \sqrt{240}}{-8}= \frac{12\pm15.5}{8}$ $\displaystyle t= \frac{-12\pm\sqrt{(12^2-4(-4)(6)}}{2(-4)}=\frac{-12\pm \sqrt{240}}{-8}= \frac{12\pm15.5}{8}$

Therefore $\displaystyle t=3.4375$ or -.4375 $\displaystyle -.4375$ and since we are looking only for positive values (because we can't have negative time), 3.4375 seconds is our answer.

Report an Error

Example Question #1 : Solving Quadratic Inequalities

Solve the quadratric inequality:

$y\geq -x^2+10x-21$ $\displaystyle y\geq -x^2+10x-21$

Possible Answers:

$x\leq2 , x\geq5$ $\displaystyle x\leq2 , x\geq5$

$x\leq1 , x\geq4$ $\displaystyle x\leq1 , x\geq4$

$x\leq3 , x\geq7$ $\displaystyle x\leq3 , x\geq7$

$x\leq5 , x\geq9$ $\displaystyle x\leq5 , x\geq9$

$x\leq4 , x\geq8$ $\displaystyle x\leq4 , x\geq8$

Correct answer:

$x\leq3 , x\geq7$ $\displaystyle x\leq3 , x\geq7$

Explanation:

Factor and solve.

$y\geq -x^2+10x-21$ $\displaystyle y\geq -x^2+10x-21$

$y\leq x^2-10x+21$ $\displaystyle y\leq x^2-10x+21$

Since the equation is less than or equal to, you know the inequality will be OR, not AND.

$y\leq (x-7)(x-3)$ $\displaystyle y\leq (x-7)(x-3)$

$x\leq3$ $\displaystyle x\leq3$ or $x\geq7$ $\displaystyle x\geq7$

Report an Error

Example Question #91 : Intermediate Single Variable Algebra

Solve the following quadratic inequality:

$y\leq x^2+4x+3$ $\displaystyle y\leq x^2+4x+3$

Possible Answers:

$x\leq -3, x\geq -1$ $\displaystyle x\leq -3, x\geq -1$

$x\leq -4, x\geq -1$ $\displaystyle x\leq -4, x\geq -1$

$x\leq -2, x\geq -1$ $\displaystyle x\leq -2, x\geq -1$

$x\leq -4, x\geq -2$ $\displaystyle x\leq -4, x\geq -2$

$x\leq -3, x\geq -2$ $\displaystyle x\leq -3, x\geq -2$

Correct answer:

$x\leq -3, x\geq -1$ $\displaystyle x\leq -3, x\geq -1$

Explanation:

Factor and solve. Since the sign is less than or equal to, we know the inequality will be OR, not AND.

$y\leq x^2+4x+3$ $\displaystyle y\leq x^2+4x+3$

$y\leq (x+3)(x+1)$ $\displaystyle y\leq (x+3)(x+1)$

$x\leq-3$ $\displaystyle x\leq-3$ or $x\geq -1$ $\displaystyle x\geq -1$

Report an Error

Example Question #41 : Solving Quadratic Equations

Solve the following quadratic inequality:

$y\leq -x^2+6x+5$ $\displaystyle y\leq -x^2+6x+5$

Possible Answers:

$3-\sqrt{13}\leq x \leq 3+\sqrt{13}$ $\displaystyle 3-\sqrt{13}\leq x \leq 3+\sqrt{13}$

$3-\sqrt{10}\leq x \leq 3+\sqrt{10}$ $\displaystyle 3-\sqrt{10}\leq x \leq 3+\sqrt{10}$

$3-\sqrt{14}\leq x \leq 3+\sqrt{14}$ $\displaystyle 3-\sqrt{14}\leq x \leq 3+\sqrt{14}$

$3-\sqrt{15}\leq x \leq 3+\sqrt{15}$ $\displaystyle 3-\sqrt{15}\leq x \leq 3+\sqrt{15}$

$3-\sqrt{11}\leq x \leq 3+\sqrt{11}$ $\displaystyle 3-\sqrt{11}\leq x \leq 3+\sqrt{11}$

Correct answer:

$3-\sqrt{14}\leq x \leq 3+\sqrt{14}$ $\displaystyle 3-\sqrt{14}\leq x \leq 3+\sqrt{14}$

Explanation:

Use the quadratic formula to solve.

$y\leq -x^2+6x+5$ $\displaystyle y\leq -x^2+6x+5$

$y\geq x^2-6x-5$ $\displaystyle y\geq x^2-6x-5$

Since the inequality is greater than or equal to, we know the inequality will be AND, not OR.

$x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$ $\displaystyle x = \frac{-b \pm \sqrt{b^2-4ac}}{2a}$

a=1 $\displaystyle a=1$

b=-6 $\displaystyle b=-6$

c=-5 $\displaystyle c=-5$

$x = \frac{-(-6) \pm \sqrt{(-6)^2-4(1)(-5)}}{2(1)}$ $\displaystyle x = \frac{-(-6) \pm \sqrt{(-6)^2-4(1)(-5)}}{2(1)}$

$x= \frac{6 \pm \sqrt{56}}{2}$ $\displaystyle x= \frac{6 \pm \sqrt{56}}{2}$

$x= 3 \pm \sqrt{14}$ $\displaystyle x= 3 \pm \sqrt{14}$

$3-\sqrt{14}\leq x \leq 3+\sqrt{14}$ $\displaystyle 3-\sqrt{14}\leq x \leq 3+\sqrt{14}$

Report an Error

Example Question #1641 : High School Math

Solve:

If $\displaystyle y$ varies directly as $\displaystyle x$, and x=7 $\displaystyle x=7$ when y=21 $\displaystyle y=21$, find $\displaystyle x$ when y=-5 $\displaystyle y=-5$.

Possible Answers:

$x=\frac{-1}{3}$ $\displaystyle x=\frac{-1}{3}$

$x=\frac{-13}{3}$ $\displaystyle x=\frac{-13}{3}$

$x=\frac{-7}{3}$ $\displaystyle x=\frac{-7}{3}$

$x=\frac{-5}{3}$ $\displaystyle x=\frac{-5}{3}$

$x=\frac{-11}{3}$ $\displaystyle x=\frac{-11}{3}$

Correct answer:

$x=\frac{-5}{3}$ $\displaystyle x=\frac{-5}{3}$

Explanation:

The formula for a direct variation is:

$\frac{x_1}{x_2}=\frac{y_1}{y_2}$ $\displaystyle \frac{x_1}{x_2}=\frac{y_1}{y_2}$

Plugging in our values, we get:

$\frac{7}{x}=\frac{21}{-5}$ $\displaystyle \frac{7}{x}=\frac{21}{-5}$

21x=-35 $\displaystyle 21x=-35$

$x= \frac{-5}{3}$ $\displaystyle x= \frac{-5}{3}$

Report an Error

Example Question #1642 : High School Math

If two boxes have the same depth and capacity, the length is inversely proportional to the width. One box is $60\ cm$ $\displaystyle 60\ cm$ long and $40\ cm$ $\displaystyle 40\ cm$ wide. A second box (same depth and capacity) is $5\ cm$ $\displaystyle 5\ cm$ long. How wide is it?

Possible Answers:

$490\ cm$ $\displaystyle 490\ cm$

$460\ cm$ $\displaystyle 460\ cm$

$480\ cm$ $\displaystyle 480\ cm$

$450\ cm$ $\displaystyle 450\ cm$

$470\ cm$ $\displaystyle 470\ cm$

Correct answer:

$480\ cm$ $\displaystyle 480\ cm$

Explanation:

The formula for an indirect variation is:

$\frac{x_1}{x_2}=\frac{y_2}{y_1}$ $\displaystyle \frac{x_1}{x_2}=\frac{y_2}{y_1}$

Plugging in our values, we get:

$\frac{60\ cm}{5\ cm}=\frac{y}{40\ cm}$ $\displaystyle \frac{60\ cm}{5\ cm}=\frac{y}{40\ cm}$

$5y=2400\ cm$ $\displaystyle 5y=2400\ cm$

$y=480\ cm$ $\displaystyle y=480\ cm$

Report an Error

View Tutors

Sandra
Certified Tutor

The Texas A&M University System Office, Bachelor in Arts, Communication, General. American InterContinental University, Maste...

View Tutors

Steven
Certified Tutor

University of Waterloo, Bachelor of Science, Chemical Engineering. McMaster University, Master of Science, Chemical Engineeri...

View Tutors

Kalee
Certified Tutor

University of Toledo, Bachelor of Education, Science Teacher Education. Westminster College, Masters in Education, Educationa...

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in Houston, Physics Tutors in Atlanta, Biology Tutors in San Diego, Biology Tutors in Seattle, Calculus Tutors in Washington DC, Math Tutors in Dallas Fort Worth, Statistics Tutors in Houston, LSAT Tutors in San Francisco-Bay Area, SAT Tutors in Chicago, Algebra Tutors in Chicago

Popular Courses & Classes

SSAT Courses & Classes in Washington DC, ISEE Courses & Classes in Denver, ACT Courses & Classes in New York City, GRE Courses & Classes in Seattle, GMAT Courses & Classes in Chicago, Spanish Courses & Classes in New York City, SAT Courses & Classes in Chicago, GMAT Courses & Classes in Atlanta, Spanish Courses & Classes in Philadelphia, Spanish Courses & Classes in San Francisco-Bay Area

Popular Test Prep

GMAT Test Prep in Boston, SSAT Test Prep in San Francisco-Bay Area, ACT Test Prep in New York City, GMAT Test Prep in Washington DC, GMAT Test Prep in Atlanta, SAT Test Prep in Seattle, GMAT Test Prep in Chicago, LSAT Test Prep in Miami, SAT Test Prep in Miami, ISEE Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : Algebra II

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #341 : Algebra Ii

Example Question #91 : Intermediate Single Variable Algebra

Example Question #1 : Using The Quadratic Formula

Example Question #1 : Using The Quadratic Formula

Example Question #2 : Quadratic Formula

Example Question #1 : Solving Quadratic Inequalities

Example Question #91 : Intermediate Single Variable Algebra

Example Question #41 : Solving Quadratic Equations

Example Question #1641 : High School Math

Example Question #1642 : High School Math

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: