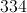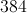Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : How to find the perimeter of a polygon

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Geometry

What is the perimeter of a regular hendecagon with a side length of 32?

Possible Answers:

334 $\displaystyle 334$

320 $\displaystyle 320$

384 $\displaystyle 384$

352 $\displaystyle 352$

Correct answer:

352 $\displaystyle 352$

Explanation:

To find the perimeter of a regular hendecagon you must first know the number of sides in a hendecagon is 11.

When you know the number of sides of a regular polygon to find the perimeter you must multiply the side length by the number of sides.

In this case it is $\displaystyle 11*32=352$.

The answer for the perimeter is 352 $\displaystyle 352$.

Report an Error

Example Question #1 : How To Find The Perimeter Of A Polygon

All segments of the polygon meet at right angles (90 degrees). The length of segment $\overline{AB}$ $\displaystyle \overline{AB}$ is 10. The length of segment $\overline{BC}$ $\displaystyle \overline{BC}$ is 8. The length of segment $\overline{DE}$ $\displaystyle \overline{DE}$ is 3. The length of segment $\overline{GH}$ $\displaystyle \overline{GH}$ is 2.

Find the perimeter of the polygon.

Possible Answers:

$\dpi{100} \small 42$ $\displaystyle \dpi{100} \small 42$

$\dpi{100} \small 46$ $\displaystyle \dpi{100} \small 46$

$\dpi{100} \small 44$ $\displaystyle \dpi{100} \small 44$

$\dpi{100} \small 40$ $\displaystyle \dpi{100} \small 40$

$\dpi{100} \small 48$ $\displaystyle \dpi{100} \small 48$

Correct answer:

$\dpi{100} \small 46$ $\displaystyle \dpi{100} \small 46$

Explanation:

The perimeter of the polygon is 46. Think of this polygon as a rectangle with two of its corners "flipped" inwards. This "flipping" changes the area of the rectangle, but not its perimeter; therefore, the top and bottom sides of the original rectangle would be 12 units long $\dpi{100} \small (10+2=12)$ $\displaystyle \dpi{100} \small (10+2=12)$. The left and right sides would be 11 units long $\dpi{100} \small (8+3=11)$ $\displaystyle \dpi{100} \small (8+3=11)$. Adding all four sides, we find that the perimeter of the recangle (and therefore, of this polygon) is 46.

Report an Error

Example Question #2 : Geometry

What is the perimeter of a regular nonagon with a side length of $\displaystyle 15$?

Possible Answers:

120 $\displaystyle 120$

150 $\displaystyle 150$

135 $\displaystyle 135$

105 $\displaystyle 105$

Correct answer:

135 $\displaystyle 135$

Explanation:

To find the perimeter of a regular polygon, we take the length of each side, $\displaystyle l$, and multiply it by the number of sides, $\displaystyle s$.

P=s*l $\displaystyle P=s*l$

In a nonagon the number of sides is $\displaystyle 9$, and in this example the side length is $\displaystyle 15$.

$\displaystyle P=9*15=135$

The perimeter is 135 $\displaystyle 135$.

Report an Error

Example Question #2 : Geometry

Find the perimeter of the following octagon:

Possible Answers:

$56\ m$ $\displaystyle 56\ m$

$96\ m$ $\displaystyle 96\ m$

$76\ m$ $\displaystyle 76\ m$

$66\ m$ $\displaystyle 66\ m$

$86\ m$ $\displaystyle 86\ m$

Correct answer:

$96\ m$ $\displaystyle 96\ m$

Explanation:

The formula for the perimeter of an octagon is $\displaystyle P = 8(side)$.

Plugging in our values, we get:

$P = 8(12\ m)$ $\displaystyle P = 8(12\ m)$

$P = 96\ m$ $\displaystyle P = 96\ m$

Report an Error

View Tutors

Messan
Certified Tutor

Indiana University-Purdue University-Indianapolis, Master of Science, Electrical Engineering. Indiana University-Purdue Unive...

View Tutors

Oksana
Certified Tutor

Khmelnytskyi Humanitarian and Pedagogical Institute, Master's/Graduate, Primary school teacher and foreign language teacher ...

View Tutors

Bobbijo
Certified Tutor

Capella University, Master of Science, Sociology.

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

LSAT Tutors in Los Angeles, MCAT Tutors in Miami, SAT Tutors in Houston, ISEE Tutors in Los Angeles, Biology Tutors in Los Angeles, ACT Tutors in New York City, GRE Tutors in Phoenix, MCAT Tutors in Dallas Fort Worth, GRE Tutors in Boston, Chemistry Tutors in Phoenix

Popular Courses & Classes

ACT Courses & Classes in Washington DC, Spanish Courses & Classes in New York City, Spanish Courses & Classes in San Diego, ACT Courses & Classes in Philadelphia, MCAT Courses & Classes in Houston, LSAT Courses & Classes in New York City, SAT Courses & Classes in Chicago, GMAT Courses & Classes in Miami, LSAT Courses & Classes in Phoenix, ACT Courses & Classes in San Diego

Popular Test Prep

ISEE Test Prep in Chicago, SAT Test Prep in Denver, ISEE Test Prep in Seattle, ISEE Test Prep in San Francisco-Bay Area, SSAT Test Prep in Chicago, SAT Test Prep in Boston, GMAT Test Prep in Chicago, GRE Test Prep in Philadelphia, SSAT Test Prep in Atlanta, SAT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : How to find the perimeter of a polygon

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #1 : Geometry

Example Question #1 : How To Find The Perimeter Of A Polygon

Example Question #2 : Geometry

Example Question #2 : Geometry

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: