ISEE Lower Level Quantitative : ISEE Lower Level (grades 5-6) Quantitative Reasoning
Study concepts, example questions & explanations for ISEE Lower Level Quantitative
All ISEE Lower Level Quantitative Resources
Example Questions
Example Question #1721 : Isee Lower Level (Grades 5 6) Quantitative Reasoning
Observe the location of the black and orange parallel lines on the provided coordinate plane and identify which of the following transformations—rotation, translation, or reflection—the black lines have undergone in order to reach the position of the orange lines. Select the answer that provides the correct transformation shown in the provided image.
A rotation
Reflection over the x-axis
A translation to the left
Reflection over the x-axis
First, let's define the possible transformations.
Rotation: A rotation means turning an image, shape, line, etc. around a central point.
Translation: A translation means moving or sliding an image, shape, line, etc. over a plane.
Reflection: A reflection mean flipping an image, shape, line, etc. over a central line.
In the images from the question, the lines were not rotated because that rotation would have caused the line to be vertical, but the line is still horizontal. The line was not moved to the left, as the translation is described in the answer choice; thus, the correct answer is a reflection over the x-axis.
Example Question #8 : Parallel Lines: Ccss.Math.Content.8.G.A.1c
Observe the location of the black and orange parallel lines on the provided coordinate plane and identify which of the following transformations—rotation, translation, or reflection—the black lines have undergone in order to reach the position of the orange lines. Select the answer that provides the correct transformation shown in the provided image.
Reflection over the y-axis
A rotation
Translation down
Translation down
First, let's define the possible transformations.
Rotation: A rotation means turning an image, shape, line, etc. around a central point.
Translation: A translation means moving or sliding an image, shape, line, etc. over a plane.
Reflection: A reflection mean flipping an image, shape, line, etc. over a central line.
In the images from the question, the lines were not rotated because that rotation would have caused the lines to be vertical, but the lines are still horizontal. The lines were not reflected over the y-axis because that transformation would have caused the orange lines to be in the top left quadrant; thus, the correct answer is a translation down.
Example Question #2 : Parallel Lines: Ccss.Math.Content.8.G.A.1c
Observe the location of the black and orange parallel lines on the provided coordinate plane and identify which of the following transformations—rotation, translation, or reflection—the black lines have undergone in order to reach the position of the orange lines. Select the answer that provides the correct transformation shown in the provided image.
A translation to the left
A rotation
Reflection over the x-axis
Reflection over the x-axis
First, let's define the possible transformations.
Rotation: A rotation means turning an image, shape, line, etc. around a central point.
Translation: A translation means moving or sliding an image, shape, line, etc. over a plane.
Reflection: A reflection mean flipping an image, shape, line, etc. over a central line.
In the images from the question, the lines were not rotated because that rotation would have moved the lines to a slant, not straight. The line was not moved to the left, as the translation is described in the answer choice; thus, the correct answer is a reflection over the x-axis.
Example Question #342 : Grade 8
Observe the location of the black and orange parallel lines on the provided coordinate plane and identify which of the following transformations—rotation, translation, or reflection—the black lines have undergone in order to reach the position of the orange lines. Select the answer that provides the correct transformation shown in the provided image.
A rotation
Reflection over the y-axis
Translation down
Translation down
First, let's define the possible transformations.
Rotation: A rotation means turning an image, shape, line, etc. around a central point.
Translation: A translation means moving or sliding an image, shape, line, etc. over a plane.
Reflection: A reflection mean flipping an image, shape, line, etc. over a central line.
In the images from the question, the lines were not rotated because that rotation would have caused the lines to be horizontal, but the lines are still vertical. The lines were not reflected over the y-axis because that transformation would have caused the orange lines to be in the top left quadrant; thus, the correct answer is a translation down.
Example Question #1722 : Isee Lower Level (Grades 5 6) Quantitative Reasoning
Observe the location of the black and orange parallel lines on the provided coordinate plane and identify which of the following transformations—rotation, translation, or reflection—the black lines have undergone in order to reach the position of the orange lines. Select the answer that provides the correct transformation shown in the provided image.
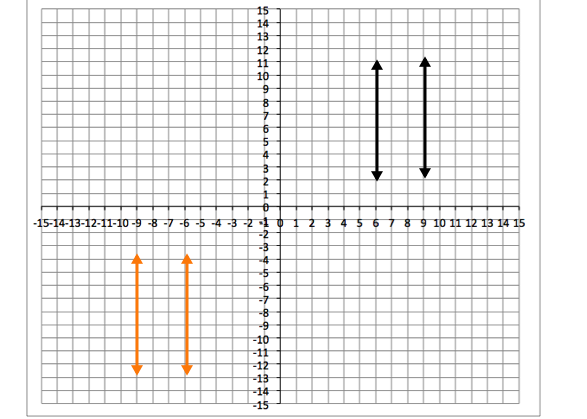
A reflection over the y-axis
A translation down and to the left
A rotation
A translation down and to the left
First, let's define the possible transformations.
Rotation: A rotation means turning an image, shape, line, etc. around a central point.
Translation: A translation means moving or sliding an image, shape, line, etc. over a plane.
Reflection: A reflection mean flipping an image, shape, line, etc. over a central line.
In the images from the question, the lines were not rotated because that rotation would have caused the lines to be horizontal, but the lines are still vertical. The lines were not reflected over the y-axis because that transformation would have caused the orange lines to be in the top left quadrant; thus, the correct answer is a translation down and to the left.
Example Question #1 : Identifying Place Value
What digit is in the hundreds place?
The hundreds place is always the third number over from the right. In this case, is the third number over from the right.
Example Question #1723 : Isee Lower Level (Grades 5 6) Quantitative Reasoning
What digit is in the tens place?
The tens place is always the second number over from the right. In this case, is the second number over from the right.
Example Question #1 : Understand Place Value For Three Digit Numbers: Ccss.Math.Content.2.Nbt.A.1
What digit is in the ones place?
The ones place is always the first number on the right. In this case, is the first number on the right.
Example Question #3 : Number & Operations In Base Ten
What digit in the hundreds place?
The hundreds place is always the third number over from the right. In this case, is the third number over from the right.
Example Question #5 : Common Core Math: Grade 2
What digit is in the tens place?
The tens place is always the second number over from the right. In this case, is the second number over from the right.
All ISEE Lower Level Quantitative Resources
