All MCAT Physical Resources
Example Questions
Example Question #4 : P H
47.0g of nitrous acid, HNO2, is added to 4L of water. What is the resulting pH?
2.0
3.2
3.5
2.5
3.0
2.0
HNO2 is a weak acid; it will not fully dissociate, so we need to use the HA → H+ + A– reaction, with ![\dpi{100} \small K_{a}=\frac{\left [ products \right ]}{\left [ reactants \right ]}=\left \frac{\left [ H^{+} \right ]\left [ A^{-} \right ]}{\left [ HA \right ]}=4.1\times 10^{-4}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/18682/gif.latex)
47.0g HNO2 is equal to 1mol. 1mol into 4L gives a concentration of 0.25M when the acid is first dissolved; however, we want the pH at equilibrium, not at the initial state. As the acid dissolves, we know [HNO2] will decrease to become ions, but we don't know by how much so we indicate the decrease as "x". As HNO2 dissolves by a factor of x, the ion concentrations will increase by x.
HNO2 → H+ + NO2–
Initial 0.25M 0 0
Equilibrium 0.25 – x x x
Now, we can fill in our equation: ![\dpi{100} \small K_{a}=\left \frac{\left [ H^{+} \right ]\left [ A^{-} \right ]}{\left [ HA \right ]}=\frac{\left ( x \right )\left ( x \right )}{0.25-x}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/18683/gif.latex)
Since x is very small, we can ignore it in the denominator:
(they expect you to do this on the MCAT; you will never have to solve with x in the denominator on the exam!)
Solve for x, and you find 
Now we can solve for pH:
Example Question #1 : P H
Acids and bases can be described in three principal ways. The Arrhenius definition is the most restrictive. It limits acids and bases to species that donate protons and hydroxide ions in solution, respectively. Examples of such acids include HCl and HBr, while KOH and NaOH are examples of bases. When in aqueous solution, these acids proceed to an equilibrium state through a dissociation reaction.
All of the bases proceed in a similar fashion.

The Brønsted-Lowry definition of an acid is a more inclusive approach. All Arrhenius acids and bases are also Brønsted-Lowry acids and bases, but the converse is not true. Brønsted-Lowry acids still reach equilibrium through the same dissociation reaction as Arrhenius acids, but the acid character is defined by different parameters. The Brønsted-Lowry definition considers bases to be hydroxide donors, like the Arrhenius definition, but also includes conjugate bases such as the A- in the above reaction. In the reverse reaction, A- accepts the proton to regenerate HA. The Brønsted-Lowry definition thus defines bases as proton acceptors, and acids as proton donors.
A scientist is studying an aqueous sample of 

The pH of the solution cannot be determined
The pH of the solution is 11
The pOH of the solution is 11
The concentration of protons is
The pH of the solution is 3
The pH of the solution is 11
Given the hydroxide ion concentration, we will need to work using pOH to find the pH. We know that the sum of pH and pOH is equal to 14.
Use our value for the concentration to find the pOH.
Now that we have the pOH, we can use it to solve for the pH.
Example Question #1 : P H
HCN dissociates based on the following reaction.
The Ka for hydrogen cyanide is 



Since HCN is a weak acid, we must use the equilibrium equation.
Because the HCN dissociates in solution, we expect the concentrations of protons and cyanide ions to increase, while the concentration of HCN will decrease. After determining the molarity of the solution, we can set up the equation below, using X as the amount of moles that dissociate.
Because X is small, we can neglect its impact in the denominator.
Since X is the concentration of protons in the solution, we can calculate the pH by using the equation ![pH = -log[H^{+}]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86307/gif.latex)
Example Question #6 : P H
There are two containers, each containing different proton concentrations. In container A, ![[H^{+} ] = 5 *10^{-6}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86294/gif.latex)
![[H^{+}] = 1*10^{-3}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/122757/gif.latex)
What is the difference in pH between these two containers?
The MCAT will typically allow you to approximate the difference in pH between two solutions. Look at the problem this way: you should already know a proton concentration of 1 * 10-3M means a pH of 3. You do not need to know the exact pH of 5 * 10-6M, but you should recognize that it is in between 1 * 10-6M and 1 * 10-5M. This means it will have a pH between 5 and 6.
The difference in pH will therefore be between 5 minus 3 and 6 minus 3.
As a result, look for the answer that is between 2 and 3. The only answer that makes sense is 2.3.
Example Question #7 : P H
An arterial blood sample from a patient has a pH of 7.4. One day later, the same patient has an arterial blood pH of 7.15. How many times more acidic is the patient's blood on the second day?
The equation to calculate pH is:
The normal pH of arterial blood is around 7.4. This reflects a concentration of hydrogen ions that can be found using the pH equation.
Using similar calculations for the second blood sample, we can find the hydrogen ion concentration again.
Now that we have both concentrations, can find the ratio of the acidity of the two samples.
You may know from biological sciences that this is approaching a lethal level of acidosis.
Example Question #61 : Acid Base Chemistry
You find a bottle in a lab that has a 
What is the pH of this solution?

Example Question #4 : Help With Buffers
Which of the following would be most useful as a buffer?
A solution of ammonia and ammonium chloride
A solution of sodium chloride and sodium hydroxide
Water
A solution of carbonic acid and sodium chloride
Potassium hydroxide
A solution of ammonia and ammonium chloride
A buffer must contain either a weak base and its salt or a weak acid and its salt. A mixture of ammonia and ammonium chloride is an example of the first case, since ammonia, NH3, is a weak base and ammonium chloride, NH4Cl, contains its salt.
Though autoionization of water produces small amounts of H3O+ and OH-, each conjugate salts of H2O, they exist in such small amounts as to make any buffering effects negligible.
Example Question #22 : Acids And Bases
Carbonic anhydrase is an important enzyme that allows CO2 and H2O to be converted into H2CO3. In addition to allowing CO2 to be dissolved into the blood and transported to the lungs for exhalation, the products of the carbonic anhydrase reaction, H2CO3 and a related compound HCO3-, also serve to control the pH of the blood to prevent acidosis or alkalosis. The carbonic anhydrase reaction and acid-base reaction are presented below.
CO2 + H2O 
H2CO3 
HCO3- may be considered a(n) __________.
intermediate
buffer compound
enzyme
second messenger
buffer compound
First, we need to determine from both the information presented in the paragraph and the chemical equation what HCO3- is doing. The paragraph tells us “HCO3- also [serves] to control the pH of the blood.” This is the definition of a buffer. A buffer is able to mitigate the addition of acid or base to a solution, or in this case, blood, by being deprotonated or protonated. For example, if the blood becomes too acidic, H+ will recombine with HCO3- to form H2CO3, thus stabilizing the pH. Alternatively, if the pH becomes too basic, H2CO3 will dissociate, releasing H+ into solution.
Example Question #1 : Buffers
HCN dissociates based on the following reaction:
The Ka for hydrogen cyanide is 
Two moles of HCN are mixed with one mole of NaCN, resulting in 1L of solution. What is the pH of the solution?
8.9
5.1
4.6
7.2
8.9
Whenever a concentration of the conjugate base is initially present in the solution, we say that the solution has been buffered. NaCN will completely dissolve in solution, meaning that there will be 1M of CN- ions in the solution initially (one mole of NaCN in 1L of soultion equals 1M). With this in mind, the new equilibrium equation for the acid's dissociation is as follows:
The initial concentrations of CN- and HCN are given in the question as 1M and 2M, respectively. The initial concentration of H+ is zero. As HCN begine to dissociate, H+ and CN- will each increase by X amount, while HCN will decrease by X amount.
Since 1M and 2M are much larger numbers than the value for X, the X values next to these numbers can be omitted from the equation.
By plugging this value into the equation ![\small pH = -log[H^{+}]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/139263/gif.latex)
This may seem wrong, because HCN is an acid, and we are used to acids resulting in solutions with pH values less than 7; however, HCN is a weak acid, and a large concentration of its conjugate base can result in a basic solution.
Example Question #24 : Acids And Bases
The pH of a buffered solution is 


In a buffered solution, when the concentrations of acid and conjugate base are equal, we know the 
This is derived from the Henderson-Hasselbalch equation: 
When concentrations are equal, the log of 


In our question, the pH is approximately one unit greater than the 
Because of this, we know that the log of the two concentrations must be equal to one.
The log of 


We can quickly narrow this question to two possible answers, since the buffered solution is more basic than the 
Certified Tutor
All MCAT Physical Resources



![\dpi{100} \small x=\left [ H^{+} \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/18686/gif.latex)
![pH=-log[H^+]=-log(1\times10^{-2})=2.0](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/83852/gif.latex)



![pOH=-\log[OH^-]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/95553/gif.latex)









![K_{a}= \frac{[CN^{-}][H^{+}]}{[HCN]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86305/gif.latex)
![\small K_{a}= \frac{[X][X]}{[[\frac{0.4mol}{2L}]-X]} = 6.2*10^{-10}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/137429/gif.latex)
![\small K_{a}= \frac{[X][X]}{[\frac{0.4mol}{2L}]} = \frac{[X]^2}{[0.2]}=6.2*10^{-10}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86306/gif.latex)

![pH=-log[1.1*10^{-5}]=4.95](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/137431/gif.latex)















![\small \left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110187/gif.latex)
![\small 7.4 = - log\left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/98016/gif.latex)
![\small 3.98 *10^{-8}M = \left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/123319/gif.latex)
![7.15=-log[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/98017/gif.latex)
![7.08*10^{-8}M=[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110188/gif.latex)
![\frac{[H^+_2]}{[H^+_1]}=\frac{7.08*10^{-8}}{3.98*10^{-8}}=1.78](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/259310/gif.latex)




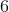

![[HNO_3]=[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/326585/gif.latex)
![pH=-log[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/326586/gif.latex)


![K_a=\frac{[H^+][A^-]}{[HA]}=\frac{[H^+][CN^-]}{[HCN]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/139261/gif.latex)
![\small 6.2*10^{-10}= \frac{[X][1+X]}{[2-X]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86313/gif.latex)
![\small 6.2*10^{-10} = \frac{[X][1]}{[2]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/139262/gif.latex)

![pH=-log[1.24*10^{-9}]=8.9](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86315/gif.latex)




![[A^-]=[HA]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/116976/gif.latex)


![pH=pK_a+log_{10}(\frac{[A^-]}{[HA]})](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/116977/gif.latex)
![pH-pK_a=1=log_{10}(\frac{[A^-]}{[HA]})](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/89686/gif.latex)
![1=log_{10}(10)=log_{10}(\frac{[A^-]}{[HA]})](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/89688/gif.latex)
![\frac{[A^-]}{[HA]}=10\rightarrow [A^-]=10[HA]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/116980/gif.latex)


