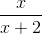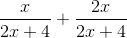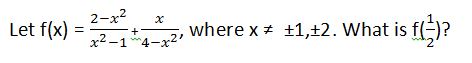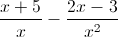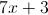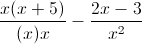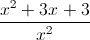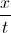Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #41 : Evaluating And Simplifying Expressions

Which of the following operations could represent the expression 2x^2+7 ?

Possible Answers:

2 times 7 less than the square of x

7 less than the square of 2x

7 more than 2 times the square of x

7 more than the square of 2x

2 times 7 more than the square of x

Correct answer:

7 more than 2 times the square of x

Explanation:

Begin by putting the equation given into your own words. It might sound something similar to:

2 times x squared plus 7

Now, go through each answer choice and see if any of them are similar to this. We immediately see that the answer "7 more than 2 times the square of x" is similar to what we came up with. Let's do a quick run through of the other choices to be sure of our choice:

"2 times 7 more than the square of x" is equal to $\small 2(x^2+7)$ , which is equivalent to $\small 2x^2+14$ .

"2 times 7 less than the square of x" is equal to $\small \small 2(x^2-7)$ , which is equivalent to $\small \small 2x^2-14$ .

"7 more than the square of 2x" is equal to $\small (2x)^2+7$ , which is equivalent to $\small 4x^2+7$ .

"7 less than the square of 2x" is equal to $\small \small (2x)^2-7$ , which is equivalent to $\small \small 4x^2-7$ .

The only answer that works is "7 more than 2 times the square of x". This is the correct answer.

Report an Error

Example Question #3 : How To Simplify An Expression

What does (-3(2+3))-4(3(2^2)) equal?

Possible Answers:

Correct answer:

Explanation:

When solving a complex expression, remember the acronym PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction). Reading left to right, begin by doing all operations within the innermost Parentheses first:

(-3(2+3))-4(3(2^2))

=(-3(5))-4(3(4))

=(-15)-4(12)

Continue simplifying using the acronym PEMDAS:

=-15-48

=-63

The expression is equal to -63.

Report an Error

Example Question #1 : Rational Expressions

Simplify.

$\frac{2x+3}{x+2}+\frac{5x-6}{x+2}$

Possible Answers:

$\frac{7x-3}{2x+4}$

$\frac{5x-1}{2}$

$\frac{7x+9}{2x+4}$

5x-1

$\frac{7x-3}{x+2}$

Correct answer:

$\frac{7x-3}{x+2}$

Explanation:

$\frac{2x-3}{x+2}+\frac{5x-6}{x+2}$

Same denominator means you add straight across the numerators, keeping the denominator the same.

$\frac{2x-3+5x-6}{x+2}$

Add like terms.

$\frac{2x+5x+3-6}{x+2}$

Final Answer.

$\frac{7x-3}{x+2}$

Report an Error

Example Question #2 : Rational Expressions

Simplify.

$\frac{x^{2}-4}{2x+8}+\frac{-12}{2x+8}$

Possible Answers:

$\frac{x-2}{6}$

$\frac{x^{2}-16}{2x+8}$

$\frac{x-4}{2}$

$\frac{x^{2}-2}{2x}$

$\frac{x^{2}-16}{x+4}$

Correct answer:

$\frac{x-4}{2}$

Explanation:

$\frac{x^{2}-4}{2x+8}+\frac{-12}{2x+8}$

Check for same Denominator

$\frac{x^{2}-4+(-12)}{2x+8}$

Add like terms

$\frac{x^{2}-16}{2x+8}$

Check for GCF or if the expression can be factored

$\frac{(x-4)(x+4)}{2(x+4)}$

After factoring, divide out like terms. x+4

$\frac{(x-4)}{2}$

Final Answer

Report an Error

Example Question #11 : Rational Expressions

Simplify the following rational expression: (9x - 2)/(x²) MINUS (6x - 8)/(x²)

Possible Answers:

$\frac{15x+6}{x}$

$\frac{12x-6}{x}$

$\frac{3x+6}{x^{2}}$

3x-10

Correct answer:

$\frac{3x+6}{x^{2}}$

Explanation:

Since both expressions have a common denominator, x², we can just recopy the denominator and focus on the numerators. We get (9x - 2) - (6x - 8). We must distribute the negative sign over the 6x - 8 expression which gives us 9x - 2 - 6x + 8 ( -2 minus a -8 gives a +6 since a negative and negative make a positive). The numerator is therefore 3x + 6.

Report an Error

Example Question #311 : Algebra

Simplify the expression.

$\frac{x}{2x+4}+\frac{x}{x+2}$

Possible Answers:

$\frac{3x}{2x+4}$

$\frac{2x}{3x+6}$

$\frac{1}{2x+4}$

$\frac{x+1}{2x+4}$

$\frac{x}{x+2}$

Correct answer:

$\frac{3x}{2x+4}$

Explanation:

To add rational expressions, first find the least common denominator. Because the denominator of the first fraction factors to 2(x+2), it is clear that this is the common denominator. Therefore, multiply the numerator and denominator of the second fraction by 2.

$\frac{x}{2x+4}+\frac{x}{x+2}$

$\frac{x}{2x+4}+\frac{(2)x}{(2)(x+2)}$

$\frac{x}{2x+4}+\frac{2x}{2x+4}$

$\frac{3x}{2x+4}$

This is the most simplified version of the rational expression.

Report an Error

Example Question #1 : Expressions

If √(ab) = 8, and a²= b, what is a?

Possible Answers:

Correct answer:

Explanation:

If we plug in a² for b in the radical expression, we get √(a³) = 8. This can be rewritten as a^3/2 = 8. Thus, log_a8 = 3/2. Plugging in the answer choices gives 4 as the correct answer.

Report an Error

Example Question #2 : Expressions

Function_part1

Possible Answers:

37/15

9/5

–11/5

–37/15

–9/5

Correct answer:

–11/5

Explanation:

Fraction_part2

Fraction_part3

Report an Error

Example Question #3 : Rational Expressions

Simplify.

$\frac{x+5}{x}-\frac{2x-3}{x^{2}}$

Possible Answers:

7x+3

$\frac{x^{2}+3x+3}{x^{2}}$

x+6

3x+3

$\frac{x^{2}+7x+3}{x^{2}}$

Correct answer:

$\frac{x^{2}+3x+3}{x^{2}}$

Explanation:

$\frac{x+5}{x}-\frac{2x-3}{x^{2}}$

Determine an LCD (Least Common Denominator) between 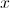and $x^{2}$ .

LCD = $x^{2}$

$\frac{x(x+5)}{(x)x}-\frac{2x-3}{x^{2}}$

Multiply the top and bottom of the first rational expression by , so that the denominator will be $x^{2}$ .

Distribute the to x+5 .

$\frac{x^{2}+5x}{x^{2}}-\frac{2x-3}{x^{2}}$

Now you can subtract because both rational expressions have the same denominators.

$\frac{x^{2}+5x-2x+3}{x^{2}}$

Final Answer.

$\frac{x^{2}+3x+3}{x^{2}}$

Report an Error

Example Question #1 : Rational Expressions

Which of the following is equivalent to $\dpi{100} \frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ ? Assume that denominators are always nonzero.

Possible Answers:

$(xt)^{-1}$

$x^{2}-t^{2}$

$x-t$

$\frac{x}{t}$

$t-x$

Correct answer:

$(xt)^{-1}$

Explanation:

We will need to simplify the expression $\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ . We can think of this as a large fraction with a numerator of $\frac{1}{t}-\frac{1}{x}$ and a denominator of $\dpi{100} x-t$ .

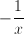
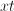
In order to simplify the numerator, we will need to combine the two fractions. When adding or subtracting fractions, we must have a common denominator. $\frac{1}{t}$ has a denominator of $\dpi{100} t$ , and $\dpi{100} -\frac{1}{x}$ has a denominator of $\dpi{100} x$ . The least common denominator that these two fractions have in common is $\dpi{100} xt$ . Thus, we are going to write equivalent fractions with denominators of $\dpi{100} xt$ .

In order to convert the fraction $\dpi{100} \frac{1}{t}$ to a denominator with $\dpi{100} xt$ , we will need to multiply the top and bottom by $\dpi{100} x$ .

$\frac{1}{t}=\frac{1\cdot x}{t\cdot x}=\frac{x}{xt}$

Similarly, we will multiply the top and bottom of $\dpi{100} -\frac{1}{x}$ by $\dpi{100} t$ .

$\frac{1}{x}=\frac{1\cdot t}{x\cdot t}=\frac{t}{xt}$

We can now rewrite $\frac{1}{t}-\frac{1}{x}$ as follows:

$\frac{1}{t}-\frac{1}{x}$ = $\frac{x}{xt}-\frac{t}{xt}=\frac{x-t}{xt}$

Let's go back to the original fraction $\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ . We will now rewrite the numerator:

$\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ = $\frac{\frac{x-t}{xt}}{x-t}$

To simplify this further, we can think of $\frac{\frac{x-t}{xt}}{x-t}$ as the same as $\frac{x-t}{xt}\div (x-t)$ . When we divide a fraction by another quantity, this is the same as multiplying the fraction by the reciprocal of that quantity. In other words, $a\div b=a\cdot \frac{1}{b}$ .

$\frac{x-t}{xt}\div (x-t)$ = $\frac{x-t}{xt}\cdot \frac{1}{x-t}$ $=\frac{x-t}{xt(x-t)}$ $= \frac{1}{xt}$

Lastly, we will use the property of exponents which states that, in general, $\frac{1}{a}=a^{-1}$ .

$\frac{1}{xt}=(xt)^{-1}$

The answer is $(xt)^{-1}$ .

Report an Error

View PSAT Mathematics Tutors

Sekou
Certified Tutor

University of New Haven, Bachelor in Arts, Mathematics.

View PSAT Mathematics Tutors

Bagher
Certified Tutor

San Jose State University, Master's/Graduate, Physics.

View PSAT Mathematics Tutors

Rodolfo
Certified Tutor

The University of Texas at San Antonio, Bachelor of Science, Mathematics.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ACT Tutors in Philadelphia, GRE Tutors in New York City, Spanish Tutors in San Diego, ISEE Tutors in Houston, Reading Tutors in Dallas Fort Worth, Physics Tutors in Boston, Calculus Tutors in Miami, Math Tutors in Washington DC, Calculus Tutors in Washington DC, SSAT Tutors in San Francisco-Bay Area

Popular Courses & Classes

GMAT Courses & Classes in Phoenix, MCAT Courses & Classes in Chicago, ACT Courses & Classes in Seattle, SSAT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Houston, ACT Courses & Classes in Boston, GMAT Courses & Classes in Chicago, ACT Courses & Classes in Houston, GRE Courses & Classes in Miami, GRE Courses & Classes in San Francisco-Bay Area

Popular Test Prep

ISEE Test Prep in Chicago, LSAT Test Prep in Denver, ISEE Test Prep in Seattle, GMAT Test Prep in Chicago, SAT Test Prep in Philadelphia, ACT Test Prep in Houston, MCAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Philadelphia, ISEE Test Prep in Boston, SAT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #41 : Evaluating And Simplifying Expressions

Example Question #3 : How To Simplify An Expression

Example Question #1 : Rational Expressions

Example Question #2 : Rational Expressions

Example Question #11 : Rational Expressions

Example Question #311 : Algebra

Example Question #1 : Expressions

Example Question #2 : Expressions

Example Question #3 : Rational Expressions

Example Question #1 : Rational Expressions

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: