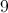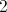All PSAT Math Resources
Example Questions
Example Question #201 : Grade 8
What is the solution to the following system of equations:
By solving one equation for 

Example Question #3 : How To Find The Solution For A System Of Equations
Jeff, the barista at Moonbucks Coffee, is having a problem. He needs to make fifty pounds of Premium Blend coffee by mixing together some Kona beans, which cost $24 per pound, with some Ethiopian Delight beans, which cost $10 per pound. The Premium Blend coffee will cost $14.20 per pound. Also, the coffee will sell for the same price mixed as it would separately.
How many pounds of Kona beans will be in the mixture?
The number of pounds of coffee beans totals 50, so one of the equations would be

The total price of the Kona beans, is its unit price, $24 per pound, multiplied by its quantity, 



We are trying to solve for 
Multiply the second equation by 

The mixture includes 15 pounds of Kona beans.
Example Question #2 : How To Find The Solution For A System Of Equations
If 


To solve this problem, you must first solve the system of equations for both 



In order to solve a system of equations, you must add the equations in a way that gets rid of one of the variables so you can solve for one variable, then for the other. One example of how to do so is as follows:
Take the equations. Multiply the first equation by two so that there is 

Add the equations:
Find the sum (notice that the variable 
Solve for 
Plug this value of 

Now, plug the values of 

The answer is 
Example Question #2 : Inequalities
Solve for 
For the second equation, solve for 

Plug this value of y into the first equation.
Example Question #514 : Basic Single Variable Algebra
Solve for 
The system has no solution
In the second equation, you can substitute 

Now, substitute 2 for 

The solution is
Example Question #2424 : Algebra 1
Without drawing a graph of either equation, find the point where the two lines intersect.
Line 1 :
Line 2 :
To find the point where these two lines intersect, set the equations equal to each other, such that 



Subtract 
Divide both sides by 2.
Now substitute 

With both coordinates, we know the point of intersection is 




Example Question #518 : Basic Single Variable Algebra
What is the sum of 

Add the equations together.
Put the terms together to see that 
Substitute this value into one of the original equaitons and solve for 
Now we know that 


Example Question #3 : How To Find The Solution For A System Of Equations
What is the solution of 
We add the two systems of equations:
For the Left Hand Side:
For the Right Hand Side:
So our resulting equation is:
Divide both sides by 10:
For the Left Hand Side:
For the Right Hand Side:
Our result is:
Example Question #264 : Algebra
What is the solution of 
Reduce the second system by dividing by 3.
Second Equation:

Then we subtract the first equation from our new equation.
First Equation:
First Equation - Second Equation:
Left Hand Side:
Right Hand Side:
Our result is:
Example Question #31 : Systems Of Equations
What is the solution of 
We first add both systems of equations.
Left Hand Side:
Right Hand Side:
Our resulting equation is:
We divide both sides by 3.
Left Hand Side:
Right Hand Side:
Our resulting equation is:
Certified Tutor
All PSAT Math Resources