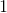First, we must order these numbers from smallest to largest.

\(\displaystyle 2,2,3,3,3,5,7,9\)

Next, subtract the smallest from the largest.

\(\displaystyle 9-2=7\)

To find the range of a function we need to find the y values that the function will cover. To do this we can create a table of values identifying the x and y values of the function.

In our case we get the following

\(\displaystyle x=0\)        \(\displaystyle y=2(0)^2+7\)         \(\displaystyle y=7\)

\(\displaystyle x=1\)        \(\displaystyle y=2(1)^2+7\)         \(\displaystyle y=9\)

\(\displaystyle x=-1\)     \(\displaystyle y=2(-1)^2+7\)     \(\displaystyle y=9\)

\(\displaystyle x=2\)        \(\displaystyle y=2(2)^2+7\)         \(\displaystyle y=15\)

\(\displaystyle x=-2\)    \(\displaystyle y=2(-2)^2+7\)      \(\displaystyle y=15\)

We see that these values great a parabola opening up and the y values at the lowest point is 7 and goes up to infinity. Therefore, the range of this function is

\(\displaystyle (7, \infty)\)

Range is the difference between the largest and smallest number in the set. In this case the smallest number is \(\displaystyle 16\) and the largest number is \(\displaystyle 103\). The difference between the two numbers is \(\displaystyle 87\).

Range is the difference between the biggest and smallest number in the set. The smallest number in the set is \(\displaystyle -23\). Remember, the bigger the negative value, the smaller it is. The largest number in the set is \(\displaystyle 35\). We take the difference. Two minus signs become a plus so our answer is \(\displaystyle 58\).

The range is the distance from the maximum number to the minimum number of a set.  

If the max goes up by \(\displaystyle 5\), then the max will be \(\displaystyle 5\) more values away from the min than it was before.  

This will increase your range by \(\displaystyle 5\).

Step 1: Arrange the numbers from smallest to largest...

\(\displaystyle \{-11,-4,2,3,7,11,23,34,71\}\)

Step 2: Subtract the smallest number FROM the largest number...

\(\displaystyle 71-(-11)\)

Step 3: Evaluate..

\(\displaystyle 71-(-11)=71+11=82\)

To find the range of a set, subtract the smallest number in the set from the largest number in the set. 

\(\displaystyle 12, 5, 7, 15,21,17,16,13,12\)

For this set, the smallest number is 5 and the largest number is 16. To solve, subtract these numbers:

\(\displaystyle 21-5=16\)

To find the range of a set, subtract the smallest number in the set from the largest number in the set

\(\displaystyle 41,38,27,42,35,38,44\)

In this case, the smallest number is 27 and the largest number is 44. To solve, subtract these numbers.

\(\displaystyle 44-27=17\)

To find the range of a set, subtract the smallest number in the set from the largest number in the set

\(\displaystyle 6,91,12,31,12\)

In this case, the smallest number is 91 and the largest number is 6. To solve, subtract these numbers.

\(\displaystyle 91-6=85\)