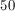Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : How to solve for a variable as part of a fraction

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #3241 : Sat Mathematics

Solve:

$\frac{4}{11}x + 3 = 15$ $\displaystyle \frac{4}{11}x + 3 = 15$

Possible Answers:

x = 29 $\displaystyle x = 29$

$x = \frac{48}{11}$ $\displaystyle x = \frac{48}{11}$

x = 37 $\displaystyle x = 37$

x = 39 $\displaystyle x = 39$

x = 33 $\displaystyle x = 33$

Correct answer:

x = 33 $\displaystyle x = 33$

Explanation:

We want to isolate the x. First, we take away 3 from both sides. Then we have:

$\frac{4}{11}x = 12$ $\displaystyle \frac{4}{11}x = 12$

To get x by itself, we multiply by the reciprocal on both sides.

Then, we have:

$x = 12 \times \frac{11}{4} = \frac{12}{1}\times\frac{11}{4} = \frac{132}{4} = 33$ $\displaystyle x = 12 \times \frac{11}{4} = \frac{12}{1}\times\frac{11}{4} = \frac{132}{4} = 33$

Report an Error

Example Question #1 : How To Solve For A Variable As Part Of A Fraction

If $\frac{6}{x}=\frac{9}{19}$ $\displaystyle \frac{6}{x}=\frac{9}{19}$ , then what is the value of $\displaystyle x$?

Possible Answers:

none of these

3/38

7/12

9/114

38/3

Correct answer:

38/3

Explanation:

cross multiply:

(6)(19) = 9x

114=9x

x = 38/3

Report an Error

Example Question #1 : How To Solve For A Variable As Part Of A Fraction

$\dpi{100} \small \frac{4 }{x} = \frac{2}{25}$ $\displaystyle \dpi{100} \small \frac{4 }{x} = \frac{2}{25}$

Find x.

Possible Answers:

$\dpi{100} \small \frac{8}{25}$ $\displaystyle \dpi{100} \small \frac{8}{25}$

$\dpi{100} \small 0.25$ $\displaystyle \dpi{100} \small 0.25$

$\dpi{100} \small \frac{25}{8}$ $\displaystyle \dpi{100} \small \frac{25}{8}$

$\dpi{100} \small 50$ $\displaystyle \dpi{100} \small 50$

None

Correct answer:

$\dpi{100} \small 50$ $\displaystyle \dpi{100} \small 50$

Explanation:

Cross multiply:

$\dpi{100} \small 4 \times 25 = 2x$ $\displaystyle \dpi{100} \small 4 \times 25 = 2x$

$\dpi{100} \small 100 = 2x$ $\displaystyle \dpi{100} \small 100 = 2x$

$\dpi{100} \small x = 50$ $\displaystyle \dpi{100} \small x = 50$

Report an Error

Example Question #2 : How To Solve For A Variable As Part Of A Fraction

The numerator of a fraction is the sum of 4 and 5 times the denominator. If you divide the fraction by 2, the numerator is 3 times the denominator. Find the simplified version of the fraction.

Possible Answers:

$\displaystyle 12$

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

$\displaystyle 18$

$\displaystyle 6$

$\frac{1}{3}$ $\displaystyle \frac{1}{3}$

Correct answer:

$\displaystyle 6$

Explanation:

Let numerator = N and denominator = D.

According to the first statement,

N = (D x 5) + 4.

According to the second statement, N / 2 = 3 * D.

Let's multiply the second equation by –2 and add itthe first equation:

–N = –6D

+[N = (D x 5) + 4]

–6D + (D x 5) + 4 = 0

–1D + 4 = 0

D = 4

Thus, N = 24.

Therefore, N/D = 24/4 = 6.

Report an Error

Example Question #11 : How To Solve For A Variable As Part Of A Fraction

Solve for x:

$\frac{x-67}{-x+85}=\frac{3}{6}$ $\displaystyle \frac{x-67}{-x+85}=\frac{3}{6}$

Possible Answers:

$\frac{9}{657}$ $\displaystyle \frac{9}{657}$

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

657 $\displaystyle 657$

$\frac{9}{1}$ $\displaystyle \frac{9}{1}$

$\displaystyle 73$

Correct answer:

$\displaystyle 73$

Explanation:

In order to solve for x, you must cross multiply the ratio first. They will be equal to each other. $\displaystyle 6(x-67)=3(-x+85)$

$\displaystyle 6x-402=-3x+255$, you then can add 3x to both sides and 402 to both sides as well. You are left with:

9x=657 $\displaystyle 9x=657$ divide both sides by 9 to get the final answer:

x=73 $\displaystyle x=73$

Report an Error

View SAT Mathematics Tutors

Noah
Certified Tutor

Bard College, Bachelors, Philosophy and Creative Writing (Dual Major). Sarah Lawrence College, Master of Fine Arts, Creative ...

View SAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

View SAT Mathematics Tutors

Paulkey
Certified Tutor

National Chiao Tung University, Bachelors, Computer Science & Information Engineering. University of Maryland Eastern Shore, ...

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

SAT Courses & Classes in Houston, SAT Courses & Classes in Phoenix, SAT Courses & Classes in San Diego, GMAT Courses & Classes in Boston, GMAT Courses & Classes in Los Angeles, Spanish Courses & Classes in Los Angeles, ACT Courses & Classes in Phoenix, Spanish Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Chicago, ACT Courses & Classes in New York City

Popular Test Prep

LSAT Test Prep in Boston, GRE Test Prep in Los Angeles, LSAT Test Prep in San Diego, GRE Test Prep in Philadelphia, MCAT Test Prep in San Francisco-Bay Area, ACT Test Prep in Chicago, SSAT Test Prep in Seattle, ACT Test Prep in New York City, GRE Test Prep in Miami, ISEE Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : How to solve for a variable as part of a fraction

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #3241 : Sat Mathematics

Example Question #1 : How To Solve For A Variable As Part Of A Fraction

Example Question #1 : How To Solve For A Variable As Part Of A Fraction

Example Question #2 : How To Solve For A Variable As Part Of A Fraction

Example Question #11 : How To Solve For A Variable As Part Of A Fraction

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: