All SSAT Upper Level Math Resources
Example Questions
Example Question #3 : How To Find The Length Of The Side Of A Right Triangle
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #4 : How To Find The Length Of The Side Of A Right Triangle
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #5 : How To Find The Length Of The Side Of A Right Triangle
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #6 : How To Find The Length Of The Side Of A Right Triangle
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #681 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #682 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.
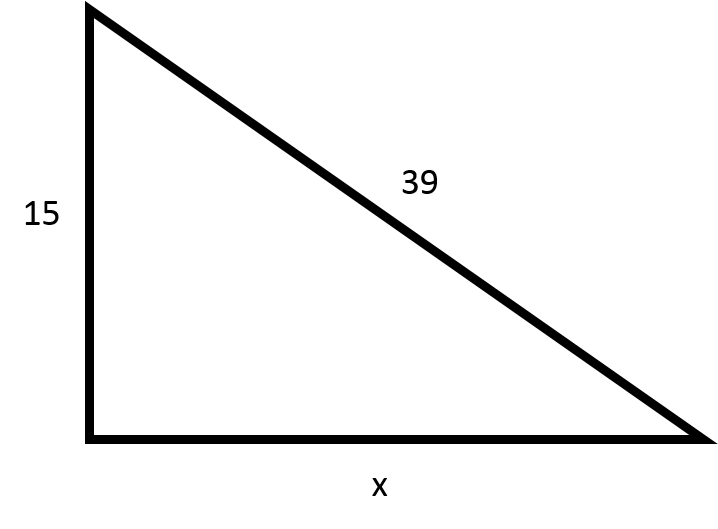
Use the Pythagorean Theorem to find the length of the missing side.
Example Question #683 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #684 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #685 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
Example Question #686 : Ssat Upper Level Quantitative (Math)
Find the length of the missing side.

Use the Pythagorean Theorem to find the length of the missing side.
All SSAT Upper Level Math Resources




















































































