All ACT Math Resources
Example Questions
Example Question #2911 : Act Math
Jane bought 30 pens, 9 of which had black ink, 10 blue ink and 11 red ink. On her way home from the store all the pens got mixed together and 2 blue pens were lost. When Jane arrives home she reaches into the bag and randomly picks a pen. What is the probability that she picks a blue pen?
When Jane starts she has a total of 30 pens, 9 black, 10 blue and 11 red. Two of the blue pens were lost. This dropped the number of blue pens to 8 and the total count of pens to 28.
To find the probability of something happening, take the total amount of things in question, the blue pens or 8, and compare them to total amount of pens which is now 28 pens.

*The pitfall for these types of questions is to realize that not only does the count of blue pens decrease but also the total count of pens. Make sure to adjust both values.
Example Question #2912 : Act Math
A spinner has the numbers 1 through 10 placed on a circle. What is the probability that the spinner lands on a number divisible by 3 on one spin?
To find the probability of an event, find the number of ways that even could happen divided by the total number of outcomes of the event.
Number of ways we could spin a number divisible by 3:
3,6,9 for 3 total
Number of different outcomes: 10.
Thus the probability is:
Example Question #83 : Probability
A coin is tossed five times. What is the probability of tails occuring at least once?
3/4
0.969
0.729
1/2
0.833
0.969
Probability of getting a head: 0.5
Probability of getting a tail: 0.5
The probability of not getting ANY tails = 0.5 * 0.5 * 0.5 * 0.5 * 0.5= 0.03125
The probability of getting AT LEAST 1 tail = 1 - 0.03125 = 0.969
Example Question #201 : Data Analysis
There are 42 candies in a jar. One-third of the candies are chocolate, one-sixth of the candies are caramel and one-sixth of candies are coconut. The rest of the candies are mint. If you select three pieces of candy from the jar, what is the approximate probability that two are chocolate and one is coconut?
2.2%
5.5%
3.5%
16.7%
1.8%
5.5%
Total Chocolates = 1/3 * 42 = 14
Total Coconuts = 1/6 * 42 = 7
There are 14 chocolates and 7 coconuts to choose from 42 total candies, and note that each time you select one candy, the total number of candies decreases by one.
So assume that to arrive at the desired outcome of two chocolate and one coconut, you chose two chocolates and then a coconut. After picking out the first chocolate, there are now 13 chocolates to choose from 41 total candies. And after picking out the second chocolate, that leaves 7 coconuts to choose from 40 total candies.
The probability of that outcome = (14/42)(13/41)(7/40) = 0.01849, or just under 1.85%
However, there are two other sequences that could arrive at that desired outcome: chocolate, coconut, chocolate (which would be 14/42 * 7/41 * 13/40 = 1.85%) and coconut, chocolate, chocolate (which would be 7/42 * 14/41 * 13/40 = 1.85%). So summing all three probabilities of favorable outcomes gives you approximately 5.5%
Example Question #1 : Trigonometry
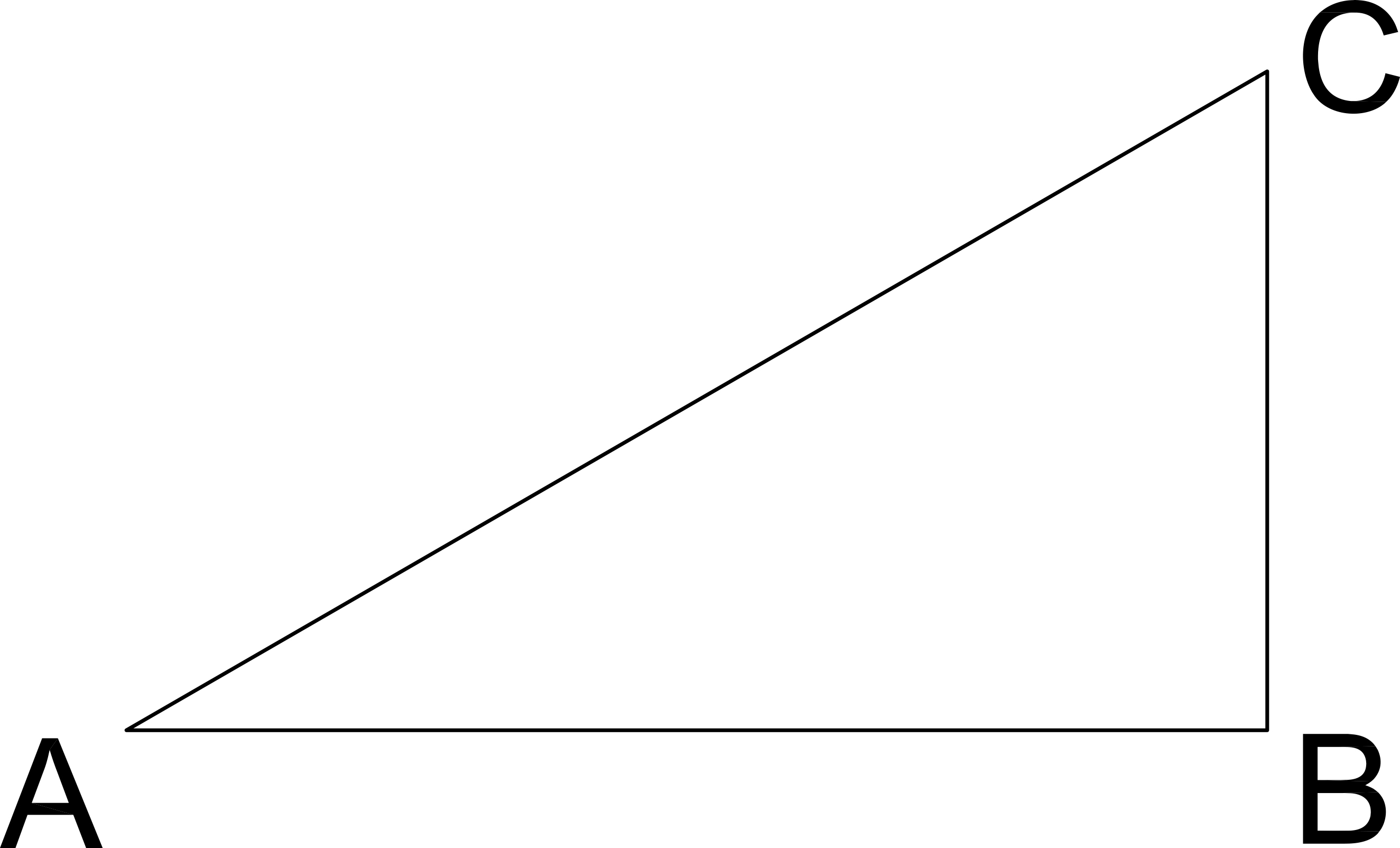
What is the tangent of C in the given right triangle??
Tangent = Opposite / Adjacent
Example Question #2913 : Act Math
Consider a right triangle with an inner angle 
If
and
what is 
The tangent of an angle x is defined as

Substituting the given values for cos x and sin x, we get

Example Question #2914 : Act Math

Triangle ABC shown is a right triangle. If the tangent of angle C is 
Use the definition of the tangent and plug in the values given:
tangent C = Opposite / Adjacent = AB / BC = 3 / 7
Therefore, BC = 7.
Example Question #4 : Trigonometry
If the sine of an angle equals 

The cosine of the angle is 


The sine of the angle equals 




Since 

Example Question #1 : How To Find An Angle With Tangent

For the above triangle, 


This triangle cannot exist.
With right triangles, we can use SOH CAH TOA to solve for unknown side lengths and angles. For this problem, we are given the opposite and adjacent sides of the triangle with relation to the angle. With this information, we can use the tangent function to find the angle.
Example Question #2 : How To Find An Angle With Tangent

In the above triangle, 


With right triangles, we can use SOH CAH TOA to solve for unknown side lengths and angles. For this problem, we are given the opposite and adjacent sides of the triangle with relation to the angle. With this information, we can use the tangent function to find the angle.
All ACT Math Resources
























































