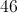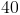All ACT Math Resources
Example Questions
Example Question #12 : Trigonometry
In a given right triangle 




In right triangles, SOHCAHTOA tells us that 




Thus, 
Example Question #9 : How To Find A Missing Side With Tangent
In a given right triangle 




In right triangles, SOHCAHTOA tells us that 




Thus, 
Example Question #13 : Trigonometry
What is the perimeter of the following figure?
The question asks for you to find the perimeter of the given figure. The figure has twelve sides total, of two varying lengths. One length is given to you, 4. The other length must be solved for using either the sine or tangent functions. However, one can arrive to answer more quickly by recognizing that the drawn triangle is actually a 3-4-5 triangle, where 3, 4, and 5 corresponds to each of the sides of the triangle. This is a pythagorean triple and this ratio should be easily remembered.
Thus if 3 is the missing side, and there are eight sides of length 3 and four sides of length 4, one can arrive to the answer:
Example Question #14 : Trigonometry
A man is setting up a laser on the ground, angling it toward the very top of a flag pole. If the flag pole is 

You can draw out your scenario like a triangle:

Now, you know that this means:
Using your calculator, you can utilize the inverse 
This rounds to 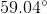
Example Question #2931 : Act Math
For the right triangle shown below, what is the value of


To solve this question, you must know SOHCAHTOA. This acronym can be broken into three parts to solve for the sine, cosine, and tangent.
We can use this information to solve our identity.
Dividing by a fraction is the same as multiplying by its reciprocal.
The sine divided by cosine is the tangent of the angle.
Example Question #1 : How To Find Positive Tangent

For triangle 

The cotangent of the angle of a triangle is the adjacent side over the opposite side. The answer is

Example Question #2 : How To Find Positive Tangent
What is the tangent of the angle formed between the origin and the point 


Recall that when you calculate a trigonometric function for an obtuse angle like this, you always use the 
Now, it is easiest to think of this like you are drawing a little triangle in the third quadrant of the Cartesian plane. It would look like:

So, the tangent of an angle is:


This is 


Example Question #3 : How To Find Positive Tangent
What is the tangent of the angle formed between the origin and the point 


Recall that when you calculate a trigonometric function for an obtuse angle like this, you always use the 
Now, it is easiest to think of this like you are drawing a little triangle in the third quadrant of the Cartesian plane. It would look like:

So, the tangent of an angle is:




Example Question #3 : How To Find Positive Tangent
A ramp is being built at an angle of 

Based on our information, we can draw this little triangle:

Since we know that the tangent of an angle is 
This can be solved using your calculator:

Now, to solve for 






Substituting in the known values:



Example Question #2933 : Act Math
What is the tangent of the angle formed between the origin and the point 


You can begin by imagining a little triangle in the second quadrant to find your reference angle. It would look like this:

The tangent of an angle is:
For our data, this is:
Now, since this is in the second quadrant, the value is negative, given the periodic nature of the tangent function.
All ACT Math Resources