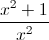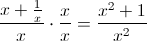All ACT Math Resources
Example Questions
Example Question #1403 : Act Math
Simplify:
Rewrite 
Change the division sign to a multiplication sign by flipping the 2nd term and simplify.
Example Question #2 : Complex Fractions
Evaluate:
The expression 
Change the division sign to a multiplication sign and take the reciprocal of the second term. Evaluate.

Example Question #2 : Complex Fractions
Simplify:
The expression 
We can simplify each fraction by multiplying the numerator by the reciprocal of the denominator.
From here we add our two new fractions together and simplify.
Example Question #26 : Fractions
Simplify the following:
Begin by simplifying your numerator. Thus, find the common denominator:
Next, combine the fractions in the numerator:
Next, remember that to divide fractions, you multiply the numerator by the reciprocal of the denominator:
Since nothing needs to be simplified, this is just:
Example Question #3 : Complex Fractions
Simplify,
Convert the numerators and denominators into single fractions, then simplify.
Start by finding the lowest common denominator in both the numerator and denominator of the complex fraction.
Add fractions with like denominators.
Simplify. Divide complex fractions by multiplying the numerator by the reciprocal of the denominator.
Solve.
Example Question #1 : How To Subtract Complex Fractions
Subtract:
The least common multiple can be found by multiplying the denominators: 2, 3, and 5. The common denominator of these numbers is 30. Multiply the numerator with what was multiplied to the denominator of each term, and then solve.
Example Question #1 : How To Subtract Complex Fractions
What is 
First, simplify both sides. 







Example Question #2 : How To Subtract Complex Fractions
Simplify:
Begin by simplifying the denominator of the first fraction:
Now, remember that division of fractions is done by multiplying the numerator by the reciprocal of the denominator. Thus:
Simplify a bit:
Example Question #1 : Complex Fractions
Simplify
Simplify the complex fraction by multiplying by the complex denominator:
Example Question #1411 : Act Math
Steven purchased 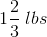

To solve this answer, we have to first make the mixed numbers improper fractions so that we can then find a common denominator. To make a mixed number into an improper fraction, you multiply the denominator by the whole number and add the result to the numerator. So, for the presented data:
and
Now, to find out how many total pounds of vegetables Steven purchased, we need to add these two improper fractions together:
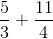
To add these fractions, they need to have a common denominator. We can adjust each fraction to have a common denominator of 




To multiply fractions, just multiply across:
We can now add the numerators together; the denominator will stay the same:
Since all of the answer choices are mixed numbers, we now need to change our improper fraction answer into a mixed number answer. We can do this by dividing the numerator by the denominator and leaving the remainder as the numerator:
This means that our final answer is 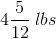
All ACT Math Resources