All Calculus 2 Resources
Example Questions
Example Question #134 : Integral Applications
What is the average value of the function 
![\left [ -2, 2\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/181080/gif.latex)
The average value of the function 
![\left [ -2, 2\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/181082/gif.latex)
Substitute 


Example Question #311 : Integrals
What is the average value of the function 
![\left [2,3 \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183519/gif.latex)
The average value of the function 
![\left [0, 1 \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183521/gif.latex)

For now, we look at the indefinite integral 



and
so
which is the antiderivative.
Now, we can find the definite integral, and, subsequently, the average value:
Example Question #131 : Integral Applications
Approximate the length of the curve of 
![[0,2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180104/gif.latex)

The length of the curve of 
![[a,b]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180107/gif.latex)





We divide the interval ![[0,2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180114/gif.latex)


By Simpson's rule, we can estimate the integral by evaluating
where 
We evaluate 
The approximation is therefore
Example Question #135 : Integral Applications
Approximate the length of the curve of 
![[0,2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180073/gif.latex)

The length of the curve of 
![[a,b]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180076/gif.latex)



We divide the interval ![[0,2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/180082/gif.latex)


By Simpson's rule, we can estimate the integral by evaluating
where
We evaluate 
The approximation is therefore
Example Question #138 : Integral Applications
What is the average value of the function 
![[0, 4]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183573/gif.latex)
The average value of the function 
![[0, 4]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183575/gif.latex)


To evaluate this integral, we note that 

![(2, 4]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183580/gif.latex)
![[0,4]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183581/gif.latex)

or
Therefore, the average value of 
Example Question #141 : Integral Applications
What is the average value of the function 
![\left [1,2 \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183494/gif.latex)
The average value of the function 
![\left [1,2 \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183496/gif.latex)

We can integrate the indefinite integral

as follows:
Set 

Then

and

Therefore,
Therefore, the average value is:
Example Question #141 : Integral Applications
What is the average value of the function 
![\left [1, e\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183473/gif.latex)
The average value of the function 
![\left [1, e\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183475/gif.latex)

Substitute 





Example Question #1 : Average Values And Lengths Of Functions
What is the arc length if 

![[0,2\pi]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/382899/gif.latex)
Write the formula for arc length.
Calculate the derivatives.
Substitute the derivatives and the bounds into the integral.
Example Question #2 : Average Values And Lengths Of Functions
What is the average value of the function

from 

The average value of a function p(t) from t=a to t=b is found with the integral

In this case, we must compute the value of the integral

A substitution makes this integral clearer. Let 

Evaluating this integral using the fact that

Example Question #3 : Average Values And Lengths Of Functions
What is the average value of the function 

In general, the average value of a function 
![[a, b]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/402701/gif.latex)
This means that the average value of 


Since the antiderivative of 


All Calculus 2 Resources




















































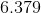



















![f(x) = \left\{\begin{matrix} -\left ( x^2 - 4\right ) & x \in [0, 2) \\ x^2 - 4 & x \in \left [ 2, 4\right ] \end{matrix}\right.](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183583/gif.latex)
![f(x) = \left\{\begin{matrix} - x^2 + 4 & x \in [0, 2) \\ x^2 - 4 & x \in \left [ 2, 4\right ] \end{matrix}\right.](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183584/gif.latex)
![\frac{1}{4 }\left [ \int_{0}^{2}\left ( - x^2 + 4 \right ) \; \mathrm{d}x + \int_{2}^{4}\left ( x^2 - 4 \right ) \; \mathrm{d}x \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183586/gif.latex)
![=\frac{1}{4 }\left [ \left.\begin{matrix} \left ( - \frac{x^3}{3} + 4x \right ) \\ \\ \end{matrix}\right| \begin{matrix} 2\\ \\ 0 \end{matrix} + \left.\begin{matrix} \left ( \frac{x^3}{3} - 4x \right ) \\ \\ \end{matrix}\right| \begin{matrix} 4\\ \\ 2 \end{matrix} \right \]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183587/gif.latex)
![=\frac{1}{4 }\left [ \left ( - \frac{2^3}{3} + 4 \cdot 2 \right ) - \left ( - \frac{0^3}{3} + 4 \cdot 0 \right ) + \left ( \frac{4^3}{3} - 4 \cdot 4\right ) - \left ( \frac{2^3}{3} - 4 \cdot 2 \right ) \right \]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183588/gif.latex)
![=\frac{1}{4 }\left [ \left ( - \frac{8}{3} + 8 \right ) -0 + \left ( \frac{64}{3} - 16\right ) - \left ( \frac{8}{3} - 8 \right ) \right \]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183589/gif.latex)
![=\frac{1}{4 }\left [ \frac{16}{3} + \frac{16}{3} - \left (- \frac{16}{3} \right ) \right \]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183590/gif.latex)



































![s=\int_{0}^{2\pi} 4 dt= 4[t]_{0}^{2\pi} = 8\pi](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/382904/gif.latex)















