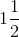All Common Core: 4th Grade Math Resources
Example Questions
Example Question #4 : Show Fractional Data On A Line Plot And Solve Problems By Using Line Plots : Ccss.Math.Content.4.Md.B.4
Use the line plot to answer the question.

Three students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.

Example Question #1037 : Common Core Math: Grade 4
Use the line plot to answer the question.

Two students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.

Example Question #1041 : Common Core Math: Grade 4
Use the line plot to answer the question.

Two students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.

Example Question #7 : Represent And Interpret Data
Use the line plot to answer the question.

Two students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.

Example Question #223 : Tables
Use the line plot to answer the question.

Four students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.
Example Question #8 : Represent And Interpret Data
Use the line plot to answer the question.

Two students spend 

When we subtract mixed numbers we subtract the whole numbers by the whole numbers and the fractions by the fractions.

Example Question #5 : Show Fractional Data On A Line Plot And Solve Problems By Using Line Plots : Ccss.Math.Content.4.Md.B.4
Use the line plot to answer the question.

Two students read 


When we add mixed numbers we add the whole numbers to the whole numbers and the fractions to the fractions.

Example Question #111 : Tables
Use the line plot to answer the question.

Three students read 

When we add mixed numbers we add the whole numbers to the whole numbers and the fractions to the fractions.
Example Question #91 : Measurement & Data
The line chart shows how many hours each student in a class spends reading each week. How many hours, on average, do the least number of students spend reading each week?

There is only 

Example Question #1 : Geometric Measurement: Understand Concepts Of Angle And Measure Angles
What is an angle that measures less than 
An obtuse angle
An acute angle
A right angle
An acute angle
By definition, an acute angle is any angle that measures less than 
All Common Core: 4th Grade Math Resources