All MCAT Physical Resources
Example Questions
Example Question #771 : Mcat Physical Sciences
The initial concentrations of this reaction are listed below.
Based on these initial concentrations, which statement is true?
The reaction will shift left
The ratio of products to reactants is greater than the equilibrium constant
The forward rate will be greater than the reverse rate
The reaction is in equilibrium
The forward rate will be greater than the reverse rate
When given initial concentrations, we can determine the reaction quotient (Q) of the reaction. The reaction quotient is given by the same equation as the equilibrium constant (concentration of products divided by concentration of reactants), but its value will fluctuate as the system reacts, whereas the equilibrium constant is based on equilibrium concentrations.
By comparing the reaction quotient to the equilibrium constant, we can determine in which direction the reaction will proceed initially.
The reaction quotient with the beginning concentrations is written below.
This shows that the ratio of products to reactants is less than the equilibrium constant. Since Q is less than Keq in the beginning, we conclude that the reaction will proceed forward until Q is equal to Keq. In this manner, the denominator (reactants) will decrease and the numerator (products) will increase, causing Q to become closer to Keq.
Example Question #8 : Equilibrium Constant And Reaction Quotient
A scientist prepares an experiment to demonstrate the second law of thermodynamics for a chemistry class. In order to conduct the experiment, the scientist brings the class outside in January and gathers a cup of water and a portable stove.
The temperature outside is –10 degrees Celsius. The scientist asks the students to consider the following when answering his questions:
Gibbs Free Energy Formula:
ΔG = ΔH – TΔS
Liquid-Solid Water Phase Change Reaction:
H2O(l) ⇌ H2O(s) + X
The scientist prepares two scenarios.
Scenario 1:
The scientist buries the cup of water outside in the snow, returns to the classroom with his class for one hour, and the class then checks on the cup. They find that the water has frozen in the cup.
Scenario 2:
The scientist then places the frozen cup of water on the stove and starts the gas. The class finds that the water melts quickly.
After the water melts, the scientist asks the students to consider two hypothetical scenarios as a thought experiment.
Scenario 3:
Once the liquid water at the end of scenario 2 melts completely, the scientist turns off the gas and monitors what happens to the water. Despite being in the cold air, the water never freezes.
Scenario 4:
The scientist takes the frozen water from the end of scenario 1, puts it on the active stove, and the water remains frozen.
The same scientist in the passage measures the variables of another reaction in the lab. He knows that this reaction is spontaneous under standard conditions, with a standard free energy change of –43 kJ/mol. Using laboratory-calculated variables, he determines that the Gibbs Free Energy has a value of 0 kJ/mol. He then calculated the reaction quotient of this reaction, while knowing the equilibrium constant was 3 x 103. What is true of the reaction quotient?
It must be one
It must be less than 3 x 103
It must be more than 3 x 103
It must be equal to 3 x 103
It must be zero
It must be equal to 3 x 103
At equilibrium, reaction quotient and equilibrium constant are equal.
Example Question #1 : Equilibrium
For any given chemical reaction, one can draw an energy diagram. Energy diagrams depict the energy levels of the different steps in a reaction, while also indicating the net change in energy and giving clues to relative reaction rate.
Below, a reaction diagram is shown for a reaction that a scientist is studying in a lab. A student began the reaction the evening before, but the scientist is unsure as to the type of the reaction. He cannot find the student’s notes, except for the reaction diagram below.

The scientist in the passage is able to calculate the reaction quotient (Q) for the reaction taking place in the vessel. If the reaction is ongoing, and has not yet reached equilibrium, how will the reaction quotient compare to the reaction constant (Keq)?
(Assume the reaction is in aqueous solution and is started with 100% reactants and no products).
Keq and Q will be equal
Keq will be less than Q
Q will be less than Keq
Q will be zero, and Keq will be greater than 1
Keq will be zero, and Q will be greater than 1
Q will be less than Keq
At equilibrium, Keq = Q. The question indicates that, starting with 100% reactants, the reaction has not yet reached equilibrium. In order to reach equilibrium, we must have a continued reduction in reactants and accumulation of products. This would necessitate an increase in Q to eventually reach the value of Keq. Essentially, Q is starting at zero and increasing to the value of Keq at equilibrium.
Example Question #1 : Analyzing Reactions
In the above reaction, by what factor would the reaction quotient change if the concentration of 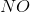
No change
For a general chemical equation 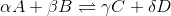
We can find the reaction quotient equation for our reaction by substituting the variables.
Notice that the concentration of 

Example Question #1 : Le Chatelier's Principle And Common Ion Effect
If the reactants and/or products in a chemical reaction are gases, the reaction rate can be determined by measuring the change of pressure as the reaction proceeds. Consider the following reaction and pressure vs. reaction rate data below.
|
Trial |
PXY(torr) |
PZ(torr) |
Rate (torr/s) |
|
1 |
100 |
200 |
0.16 |
|
2 |
200 |
200 |
0.32 |
|
3 |
200 |
100 |
0.04 |
|
4 |
200 |
150 |
0.14 |
If the volume of the container were reduced, what would happen to the rate of the reaction?
Decrease
Stay the same
Increase
It depends on the pressure
Increase
Reducing the volume of the container increases pressure. This results in a higher frequency of gas particle collisions, thereby increasing the rate of the reaction.
Example Question #1 : Le Chatelier's Principle And Common Ion Effect
Consider the following equation for the production of ammonia gas from hydrogen gas and nitrogen gas.
If the volume of the vessel containing hydrogen and nitrogen is decreased, the production of ammonia __________.
is not affected
is disfavored
depends on original volume
is favored
is favored
Since decreasing the volume of the container has the effect of increasing pressure, equilibrium is shifted to the right. An increase in pressure has the result of favoring the side of the reaction with fewer moles of gas. (According to the balanced equation, there are 4 moles on the reactant side as opposed to 2 moles on the product side).
Example Question #3 : Le Chatelier's Principle And Common Ion Effect
Barium fluoride dissolves in solution according to the following equation.
Enough BaF2 is added to create a saturated liter of aqueous solution.
Suppose that 1M NaF is added to the solution, such that it does not change the volume of the solution. What would you expect to change as a result of the addition of NaF?
More BaF2 will dissolve.
The solubility of BaF2 will decrease.
The pH of the solution will decrease.
The solubility product constant will decrease.
The solubility of BaF2 will decrease.
By adding NaF to the equation, 1M of F- ions are added to the solution. Thinking in terms of Le Chatlier's principle, the addition of a compound on one side of the equation will cause a shift to the other side of the reaction.
It DOES NOT affect the solubility product constant. Since there is an addition to the products side of the reaction, there will be a shift to the left, and more salt (reactant) will be precipitated. As a result, the solubility of the salt has decreased, because of the addition of NaF. This is known as the common ion effect.
Example Question #771 : Mcat Physical Sciences
The Haber-Bosch process, or simply the Haber process, is a common industrial reaction that generates ammonia from nitrogen and hydrogen gas. A worker in a company generates ammonia from the Haber process. He then dissociates the gaseous ammonia in water to produce an aqueous solution. Since ammonia is a base, it will accept a proton from water, generating 
The worker could do which of the following to shift the equilibrium towards hydroxide (
I. Add hydrogen gas
II. Add
III. Add
I and II
III only
I only
I and III
I and III
You need to understand Le Chatelier’s principle to solve this question. Le Chatelier’s principle states that the addition of excess molecules to one side of a reaction will shift the equilibrium toward the other side. This shift makes sure that the reaction remains at equilibrium.
If you add hydrogen gas, reaction 1 will shift to the right and will produce more ammonia. Since more ammonia is produced, reaction 2 will shift to the right to produce more ammonium ions and hydroxide ions. Statement I is correct.
Adding 
Finally, adding 



Example Question #11 : Equilibrium
The Haber-Bosch process, or simply the Haber process, is a common industrial reaction that generates ammonia from nitrogen and hydrogen gas. A worker in a company generates ammonia from the Haber process. He then dissociates the gaseous ammonia in water to produce an aqueous solution. Since ammonia is a base, it will accept a proton from water, generating 
What will happen if the worker increases the pH?
The amount of ammonium ions will increase
Changing the pH will have no effect
The amount of ammonia will stay constant
The equilibrium of reaction 2 will shift to the left
The equilibrium of reaction 2 will shift to the left
Increasing the pH is equivalent to decreasing hydrogen ion concentration or increasing hydroxide ion concentration. This increase in hydroxide ion concentration will shift the equilibrium of reaction 2 to the left, producing more ammonia. This is a fundamental point of Le Chatelier's principle.
Example Question #5 : Le Chatelier's Principle And Common Ion Effect
Two solutions of the same ionic compound are prepared. One solution uses a pure water solvent, while the other uses a solution of dilute sodium chloride. Equal amounts of the ionic compound are added to each solvent. A student observes that a precipitate forms in the solution that used brine as a solvent, but not in the aqueous solution.
What is the most likely cause of this phenomenon?
A dissociation reaction occurred
The solution is saturated
Le Chatelier’s principle
Common-ion effect
Common-ion effect
This phenomenon is called the common-ion effect. When a compound dissolves in water it dissociates into ions. Increasing the concentration of one of these ions will shift the equilibrium towards the compound, thereby making it hard for the compound to dissolve in water (decreases solubility of compound). The ionic compound may have been something like silver chloride, which has a low solubility in water. A small amount may dissolve in aqueous solution, but the preexistence of chloride ions in the solvent would reduce the already low solubility and generate a precipitate.
Increasing chloride ion concentration shifts equilibrium to the left. While this phenomenon is directly linked to Le Chatelier's principle, the direct term is the common-ion effect.
A dissociation reaction does occur, but that does not explain the formation of the precipitate in only one solution. Also, since equal amounts of the ionic compounds are added to each solvent, we cannot make any conclusions about saturation.
Certified Tutor
All MCAT Physical Resources



![[CH_{3}OH] = 2.5M, [CO] = 1.3M, [H_{2}] =0.6M.](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/115362/gif.latex)
![Q=\frac{[CO][H_2]^2}{[CH_3OH]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183371/gif.latex)
![Q\ \text{or}\ K_{eq}=\frac{[CH_3OH]}{[H_2]^2[CO]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/183372/gif.latex)
![\frac{[2.5]}{[0.6]^{2}[1.3]} = 5.3](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/86003/gif.latex)
![Q=\frac{[products]}{[reactants]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110330/gif.latex)
![K_{eq}=\frac{[equilibrium\ product]}{[equilibrium\ reactant]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/85249/gif.latex)
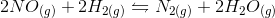




![Q=\frac{[C]^{\gamma}[D]^{\delta}}{[A]^{\alpha}[B]^{\beta}}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/668140/gif.latex)

![Q=\frac{[N_{2}][H_{2}O]^{2}}{[NO]^{2}{[H_{2}]}^{2}}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/668141/gif.latex)
![Q_2=\frac{[N_{2}][H_{2}O]^{2}}{[2NO]^{2}{[H_{2}]}^{2}}=\frac{[N_{2}][H_{2}O]^{2}}{4[NO]^{2}{[H_{2}]}^{2}}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/668142/gif.latex)















