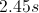In order to solve for the amount of time, we need to know the vertical initial velocity of the cannonball. This is given by the equation . Using the initial velocity of  we determine that the vertical initial velocity is 

Knowing this, we can solve the amount of time it will take for the cannon ball to reach its maximum peak, with a velocity of  using the equation 

This is the amount of time it takes for the ball to reach its peak, not the total time it is in the air. Since the ball must drop from the maximum height, we double the time and arrive at the correct answer of 20.8s.

The only acceleration on the cannon ball is done by gravity, which is constant throughout the ball's flight. Since there is no air resistance, the horizontal velocity of the ball is also constant, however, the vertical velocity of the ball is not constant, because the velocity of the ball changes throughout its flight.

It is  at the beginning ()and is  at the maximum height of the cannon ball.

The velocity must be broken down into x (horizontal) and y (vertical) components. We can use the y component to find how high the object gets. To find vertical velocity, v_y, use .

Next we find how long it takes to reach the top of its trajectory using .

 t = 5.3s

Finally, find how high the object goes with .

First, find the horizontal (x) and vertical (y) components of the velocity

Next, find how long the object is in the air by calculating the time it takes it to reach the top of its path, and doubling that number.

t = 6.25s 

Total time in the air is therefore 12.5s (twice this value).

Finally, find distance traveled my multiplying horizontal velocity and time.

We can start this problem by determining how much time it takes the box the reach the ground. Since the vertical distance is 30m and we know the angle of the ramp.

Now that we have the distance traveled, we can determine the acceleration on the box due to gravity, using the equation .

We can plug these values into the following distance equation and solve for time.

Now that we know the acceleration on the box and the time of travel, we can use the equation  to solve for the velocity.

We can start this problem by determining how far the box will travel on the ramp before hitting the ground. Since the vertical distance is 30m and we know the angle of the ramp, we can determine the length of the hypotenuse using the equation .

Now that we have the distance traveled, we can determine the acceleration on the box due to gravity. Because the box is on a sloped surface, the box will not experience the full acceleration of gravity, but will instead be accelerated at a value of . Since the angle is 60^o, the acceleration on the box is  

Finally, we can plug these values into the following distance equation and solve for time.

We must first determine the amount of time that the ball is in the air, then use the horizontal velocity to solve for the distance travelled.

In order to solve for the amount of time, we need to know the vertical initial velocity of the cannonball. This is given by the equation . Using the initial velocity of  we determine that the vertical initial velocity is 

Knowing this, we can solve the amount of time it will take for the cannon ball to reach its maximum peak, with a velocity of  using the equation 

This is the amount of time it takes for the ball to reach its peak, not the total time it is in the air. Since the ball must drop from the maximum height, we double the time and find that total flight time is 20.8s.

The next step is determining the horizontal initial velocity from the cannon. This is determined using the equation below.

Since we assume that there is no air resistance, we can conclude that the horizontal velocity of the cannonball will remain constant throughout its flight. As a result, the total displacement equation is simplified to the following: .

First, solve for the initial vertical velocity of the ball.

v_y = (10m/s)(sin(45^o)) = 7.1m/s

Think back to the 3 main kinematics equations we know:

v_f² = v_i² + 2aΔx

v_f = v_i + at

Δx = v_it + ½at²

We need to choose an equation that allows us to solve for the vertical height of the ball, given that we know the initial vertical velocity, final vertical velocity (zero), and the acceleration due to gravity (-9.8m/s²).

v_f² = v_i² + 2aΔy

Δy = (v_f² - v_i²)/2a

Once again, think back to our 3 main kinematics question:

v_f²  = v_i² + 2aΔx

v_f = v_i + at

Δx = v_it + ½at²

We want an equation that allows us to determine the time the ball spends in flight. Often, it is easier to compute the time it takes for the ball to reach its maximum height, then multiply by two. However, this “shortcut” only works when the launch point and end point are at the same vertical height, as they are in this problem.

v_f = v_i + at

t = (v_f – v_i)/a

Remember to use the veritcal velocity as your initial velocity.

v_y = (10m/s)(sin(45^o)) = 7.1m/s

This is one half of the flight time. We multiply by two to find the total time.

t = 2(0.72s) = 1.44s

First, we will need the time that the ball is in flight.

Think back to our 3 main kinematics question:

v_f²  = v_i² + 2aΔx

v_f = v_i + at

Δx = v_it + ½at²

We want an equation that allows us to determine the time the ball spends in flight. Often, it is easier to compute the time it takes for the ball to reach its maximum height, then multiply by two. However, this “shortcut” only works when the launch point and end point are at the same vertical height, as they are in this problem.

v_f = v_i + at

t = (v_f – v_i)/a

Remember to use the veritcal velocity as your initial velocity.

v_y = (10m/s)(sin(45^o)) = 7.1m/s

This is one half of the flight time. We multiply by two to find the total time.

t = 2(0.72s) = 1.44s

Using this time with the initial horizontal velocity will allow us to solve for the total horizontal distance.

 v_x = (10m/s)(cos(45^o)) = 7.1m/s

Δx = v_it + ½at²

Δx = (7.1m/s)(1.44s) = 10.22m