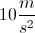This question asks us to consider gravitational potential energy. We are provided the mass of the ball (2kg) and know the acceleration due to gravity (9.8m/s²). Using the height of the ball at the top of its arc, we can solve for gravitational potential energy.

First, solve for the initial vertical velocity of the ball.

v_y = (10m/s)(sin(45^o)) = 7.1m/s

Think back to the 3 main kinematics equations we know:

v_f² = v_i² + 2aΔx

v_f = v_i + at

Δx = v_it + ½at²

We need to choose an equation that allows us to solve for the vertical height of the ball, given that we know the initial vertical velocity, final vertical velocity (zero), and the acceleration due to gravity (-9.8m/s²).

v_f² = v_i² + 2aΔy

Δy = (v_f² - v_i²)/2a

Now that we know the maximum height, we can find the potential energy from this position.

U = mgh

U = (2kg)(9.8m/s²)(2.57m) = 50.4J

To find the acceleration of the box traveling down the incline, the mass is not needed. Using the incline of the plane as the x-direction, we can see that there is no movement in the y-direction; therefore, we can use Newton's second, F = ma, in the x-direction.

There is only one force in the x-direction (gravity), however gravity is not just equal to “mg” in this case. Since the box is on an incline, the gravitational force will be equal to mgsin(30^o). Substituting force into F  =ma we find that mgsin(30^o) = ma. We can now cancel out masses and solve for acceleration.

Consider a triangle in which the initial velocity is the hypotenuse. The horizontal component of the velocity can be found using trigonometry:

Use our given values to solve:

Displacement is the change in from intial position to final position. The car first travels 30 miles east and then returns 3 miles west.

East:

West:

They have traveled 27 miles east from their initial position.

The force of gravity on the box will be in the downward direction, but the acceleration generated will be parallel to the surface of the incline. We can break the total force of gravity into parallel (horizontal) and perpendicular (vertical) components.

Calculate the horizontal force on the box.

Now that we know the horizontal force, we can solve for the horizontal acceleration by using the mass of the box and Newton's second law.

The counterclockwise and clockwise torques about the pivot point must be equal for the rod to balance. Taking the fulcrum as the pivot point, the counterclockwise torque is due to the rod’s weight, gravitational force acting downwards at the center of the rod. If we use the pivot as our reference, then the center of the rod is 15cm from the reference.

Set this equal to the clockwise torque due to the additional mass, a distance r to the right of the pivot.

.

Solving for r gives r = 0.05m to the right of the pivot, so 40 + 5 cm from the left end of the rod.

The net torque on the pulley is zero. Remember that , assuming the force acts perpendicular to the radius. Because the pulley is symmetrical in this problem (meaning the r is the same) and the tension throughout the entire rope is the same (meaning F is the same), we know that the counterclockwise torque cancels out the clockwise torque, thus, the net torque is zero.

In the image below, T₁ (due to the platform with the 4 0.5kg weights) = T₂ (the 2kg mass).

Torque is defined by the equation . Since both students will exert a downward force perpendicular to the length of the seesaw, . In our case, force is the force of gravity, given below, and is the distance from the center of the seesaw.

Since the torque must be zero in order for the seesaw to stay parallel (not move), the lighter student on the right must make his torque on the right equal to the torque of the student on the left. We can determine the required distance by setting their torques equal to each other.

Torque, in this case, is dependent on both the force exerted by the students as well as their distances from the point of rotation. As a result, both students moving forward by one meter will cause a nonzero torque on the seesaw. This is because the heavier student's ratio of force and distance will result in less torque on his side than the lighter student.

This a an example of rotational equilibrium involving torque. The formula for torque is , where  is the angle that the force vector makes with the object in equilibrium and  is the distance from the fulcrum to the point of the force vector. To achieve equilibrium, our torques must be equal.

Since the forces are applied perpendicular to the beam, becomes 1. The distance of the fulcrum from the left end is 1m and its distance from the right end is 2m.

Since the 50N force is twice as far from the fulcrum as the force that must be applied on the left side, it must be half as strong as the force on the left. The force on the left can be found to be 100N.